
A rectangle is inscribed in an equilateral triangle of side length 2a units. The maximum area of this rectangle can be
a.
b.
c.
d.
Answer
525k+ views
Hint: Proceeding the question by first drawing the figure, we try to evaluate the area of the rectangle inscribed in the equilateral triangle in terms of only one variable. Then we will differentiate the obtained area to get the value of the variable at which the area is maximum.
Complete step-by-step answer:
First of all, we will draw the figure of the given question.
We draw an equilateral triangle ABC and inscribe a rectangle inside it given by DEFG, where the side D meets line AB, E meets line AC and F and G meet line BC of the equilateral triangle.
Let the length of line BC be 2a, because the given equilateral triangle is of side 2a and let the length of line FG be l then the remaining part of the line BC becomes
BF = GC =
Using all these above calculations and assumptions we obtain the figure as ,
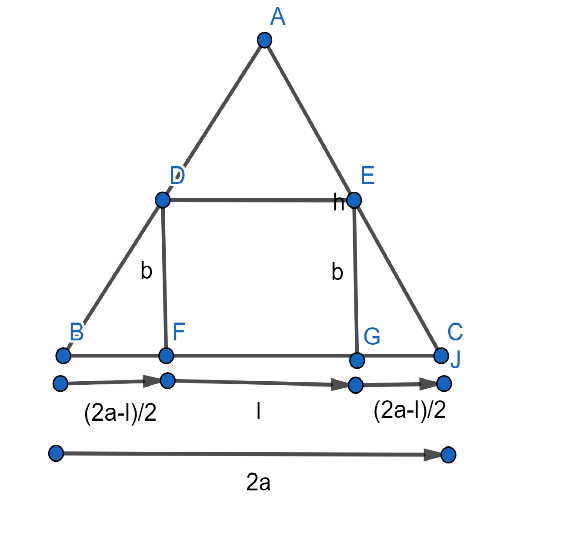
Now consider the triangle DBF,
Using trigonometric properties of tan and applying tan on triangle DBF we get,
We have value of tan 60 =
Now as DEFG is a rectangle and area of the rectangle is lb, where l is the length and b is breadth of the rectangle.
Therefore, Area of rectangle DEFG = l×b
We earlier obtained breadth of the rectangle as
Then the area of rectangle DEFG becomes
For maximum area we evaluate
We have,
Differentiating the above with respect to l we get,
Equating
Therefore, Maximum Area = l×b will be obtained by substituting a=l in area A and breadth b.
Hence, maximum area is
Hence, we have Maximum Area
Note: The possibility of error in the question is at the point where we try to calculate the area of the rectangle inscribed in the equilateral triangle. Always try to obtain area in one single variable rather than going for multiple variables. This helps while differentiating it as then we do not have to rely only on one variable used in an area.
Complete step-by-step answer:
First of all, we will draw the figure of the given question.
We draw an equilateral triangle ABC and inscribe a rectangle inside it given by DEFG, where the side D meets line AB, E meets line AC and F and G meet line BC of the equilateral triangle.
Let the length of line BC be 2a, because the given equilateral triangle is of side 2a and let the length of line FG be l then the remaining part of the line BC becomes
BF = GC =
Using all these above calculations and assumptions we obtain the figure as ,

Now consider the triangle DBF,
Using trigonometric properties of tan and applying tan on triangle DBF we get,
We have value of tan 60 =
Now as DEFG is a rectangle and area of the rectangle is lb, where l is the length and b is breadth of the rectangle.
Therefore, Area of rectangle DEFG = l×b
We earlier obtained breadth of the rectangle as
Then the area of rectangle DEFG becomes
For maximum area we evaluate
We have,
Differentiating the above with respect to l we get,
Equating
Therefore, Maximum Area = l×b will be obtained by substituting a=l in area A and breadth b.
Hence, maximum area is
Hence, we have Maximum Area
Note: The possibility of error in the question is at the point where we try to calculate the area of the rectangle inscribed in the equilateral triangle. Always try to obtain area in one single variable rather than going for multiple variables. This helps while differentiating it as then we do not have to rely only on one variable used in an area.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




