
A right circular cylinder having a diameter 12cm and height 15cm is full of ice-cream. The ice-cream is to be filled in cones of height 12cm and diameter 6cm having a hemispherical shape on the top. Find the number of such cones which can be filled with ice-cream.
Answer
512.7k+ views
Hint: Here, first we have to find the volume of the cylinder using the formula
Complete step-by-step answer:
We are given a right circular cylinder full of ice-cream. The height and diameter of the cylinder are,
Therefore, radius
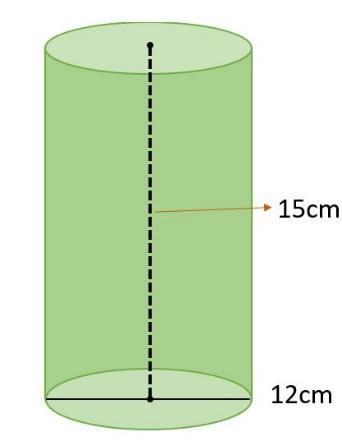
Next, we have to find the volume of the cylinder,
Hence, the volume of the cylinder is given by
Next, we have to fill the ice-cream in a cone having a hemispherical shape on the top.
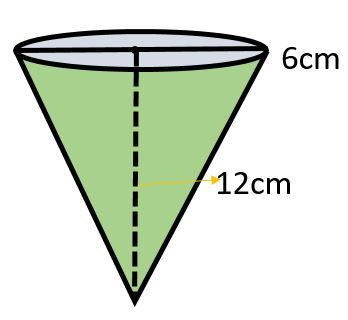
The height and diameter of the cone is given as,
Therefore, the radius
Next, we have to find the volume of the cone. The volume of the cone
Hence, the volume of the cone is given by,
Since the cone has a hemispherical top, the diameter of the cone is the same as the diameter of the hemisphere.
Therefore, the diameter of the hemisphere is given by
That is, the radius of the hemisphere
Next, we have to find the volume of the hemisphere. The volume of the hemisphere
Hence, the volume of the hemisphere is given by,
Now, we have to find the number of cones which can be filled with ice-cream.
Number of ice-cream cones, n is obtained by:
By cancellation, we get,
Therefore, the number of cones that can be filled with ice-cream is 10.
Note: Here, we have to calculate the number of ice-cream filled cones, so while considering cones you have to take, volume of cone + volume of hemisphere, don’t take the volume of cone alone which may lead to a wrong answer.
Complete step-by-step answer:
We are given a right circular cylinder full of ice-cream. The height and diameter of the cylinder are,
Therefore, radius

Next, we have to find the volume of the cylinder,
Hence, the volume of the cylinder is given by
Next, we have to fill the ice-cream in a cone having a hemispherical shape on the top.

The height and diameter of the cone is given as,
Therefore, the radius
Next, we have to find the volume of the cone. The volume of the cone
Hence, the volume of the cone is given by,
Since the cone has a hemispherical top, the diameter of the cone is the same as the diameter of the hemisphere.
Therefore, the diameter of the hemisphere is given by
That is, the radius of the hemisphere
Next, we have to find the volume of the hemisphere. The volume of the hemisphere
Hence, the volume of the hemisphere is given by,
Now, we have to find the number of cones which can be filled with ice-cream.
Number of ice-cream cones, n is obtained by:
By cancellation, we get,
Therefore, the number of cones that can be filled with ice-cream is 10.
Note: Here, we have to calculate the number of ice-cream filled cones, so while considering cones you have to take, volume of cone + volume of hemisphere, don’t take the volume of cone alone which may lead to a wrong answer.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

What is the feminine gender of a stag class 8 english CBSE

Give me the opposite gender of Duck class 8 english CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE




