
A right cone is
Answer
521.7k+ views
Hint: The given question is related to the volume of cones. The volume of the cone before and after melting and recasting will be the same. To find the height of the new cone, first, find the volume of the first cone. Then, assume the height of the new cone as
Complete step-by-step answer:
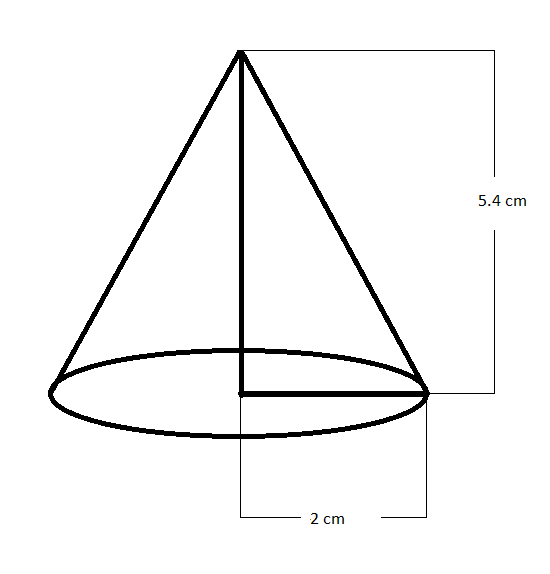
We are given the initial height of the cone as
Now, we do not know the height of the new cone. So, let’s assume it to be equal to
Now, we are given that the initial cone is melted and recast into the new cone. So, their volumes must be equal, i.e.
So,
Hence, the height of the new cone is
Note: While solving problems related to areas and volumes, always make sure that the units of the dimensions are the same. If the units are not the same then, use the unit conversion relations to make the units same. Mathematical operations cannot be performed between two entities with different units. Students generally do not care for the units and make calculations without ensuring that the units are the same and end up getting a wrong answer.
Complete step-by-step answer:

We are given the initial height of the cone as
Now, we do not know the height of the new cone. So, let’s assume it to be equal to
Now, we are given that the initial cone is melted and recast into the new cone. So, their volumes must be equal, i.e.
So,
Hence, the height of the new cone is
Note: While solving problems related to areas and volumes, always make sure that the units of the dimensions are the same. If the units are not the same then, use the unit conversion relations to make the units same. Mathematical operations cannot be performed between two entities with different units. Students generally do not care for the units and make calculations without ensuring that the units are the same and end up getting a wrong answer.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

What is the feminine gender of a stag class 8 english CBSE

Give me the opposite gender of Duck class 8 english CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE





