
A ring of mass

Answer
477k+ views
Hint: The kinetic energy of the system is given and we are asked to find the value of x. To find the value of x we have to calculate the kinetic energy of the system. For this, first find the kinetic energy of each particle and then kinetic energy of the ring and equate the kinetic energy of the system obtained with the given value.
Complete step by step answer:
Given, velocity of centre is
As the velocity of the centre is
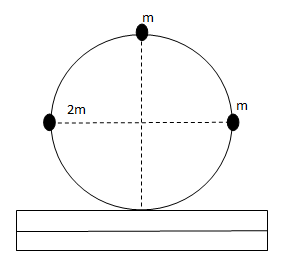
Let us draw a diagram,

Let O be the centre and A be the point of contact between ring and block,
Now, let us find the kinetic energy of each particle.
For particle 1, let
We have,
Kinetic energy of particle 1 with mass
For particle 2, let
Kinetic energy of particle 2 with mass
For particle 3, let
Kinetic energy of particle 3 with mass
Therefore, total kinetic energy of the particles
Kinetic energy of ring is,
Now, the rotational kinetic energy of the ring is,
Here ,
We have the formula for moment of inertia of a ring about an axis passing through its centre as,
Now, putting the value of
And translational kinetic energy of the ring is
Therefore, kinetic energy of the ring
Now, kinetic energy of the system is,
Putting the values of
We are given that kinetic energy of the system is
So, comparing this value with our calculated value we get,
Thus, the value of
Note:
In such types of questions where there is more than one particle, first try to find the energies of each particle. And look carefully for all the clues given in the question, like here we were given slipping is absent which makes the problem simple as that means the velocity of the contact point is zero. So, for such questions apply all the conditions given in the questions this will simplify the problem.
Complete step by step answer:
Given, velocity of centre is
As the velocity of the centre is
Let us draw a diagram,

Let O be the centre and A be the point of contact between ring and block,
Now, let us find the kinetic energy of each particle.
For particle 1, let
We have,
Kinetic energy of particle 1 with mass
For particle 2, let
Kinetic energy of particle 2 with mass
For particle 3, let
Kinetic energy of particle 3 with mass
Therefore, total kinetic energy of the particles
Kinetic energy of ring is,
Now, the rotational kinetic energy of the ring is,
Here ,
We have the formula for moment of inertia of a ring about an axis passing through its centre as,
Now, putting the value of
And translational kinetic energy of the ring is
Therefore, kinetic energy of the ring
Now, kinetic energy of the system is,
Putting the values of
We are given that kinetic energy of the system is
So, comparing this value with our calculated value we get,
Thus, the value of
Note:
In such types of questions where there is more than one particle, first try to find the energies of each particle. And look carefully for all the clues given in the question, like here we were given slipping is absent which makes the problem simple as that means the velocity of the contact point is zero. So, for such questions apply all the conditions given in the questions this will simplify the problem.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




