
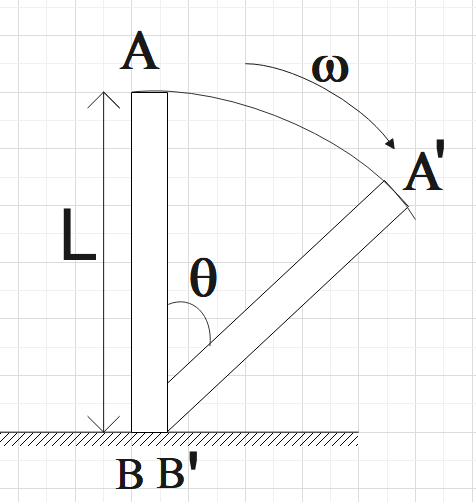
A rod is free to rotate about the Y axis as shown. It begins to rotate from its unstable equilibrium position. When it has turned through an angle
A.
B.
C.
D.

Answer
421.8k+ views
Hint: As a very first step, one could read the question and find that the system’s total mechanical energy is conserved. Now you could find that the change of centre of gravity takes place and hence you could find the related change in potential energy and then equate it to the kinetic energy and hence find the answer.
Complete step by step solution:
In the question, we are given a uniform rod of length l that is free to rotate in a vertical plane about a certain fixed axis through B. The rotation of the rod begins from its unstable equilibrium position. We are supposed to find the angular velocity
Since only the weight of the body, which is a conservative force acts on the rod, the total mechanical energy will be conserved.
Mechanical energy will be the sum of kinetic and potential energy.
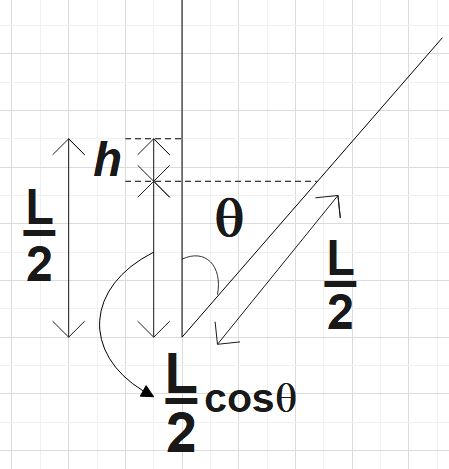
So, the above figure gives the height h through which the centre of gravity has shifted. From the figure we find this length to be,
The change in potential energy would be,
Rotational kinetic energy would be given by,
Where, moment of inertia of a rod would be,
We could equate (2) and (3) as the system is conservative,
Therefore, we found the angular velocity to be,
Hence, option B is correct.
Note: Now, you may be wondering why haven’t we discussed anything about the translational kinetic energy. This is simply because it just doesn’t exist here. You may find that the point at which the rod is hinged doesn’t move and is fixed and thereby we shouldn’t worry about the translational motion and also the related kinetic energy.
Complete step by step solution:
In the question, we are given a uniform rod of length l that is free to rotate in a vertical plane about a certain fixed axis through B. The rotation of the rod begins from its unstable equilibrium position. We are supposed to find the angular velocity
Since only the weight of the body, which is a conservative force acts on the rod, the total mechanical energy will be conserved.
Mechanical energy will be the sum of kinetic and potential energy.
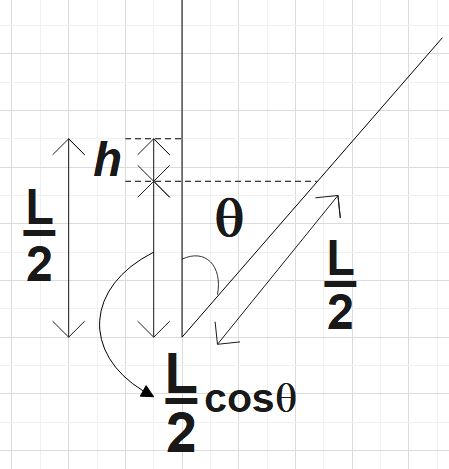
So, the above figure gives the height h through which the centre of gravity has shifted. From the figure we find this length to be,
The change in potential energy would be,
Rotational kinetic energy would be given by,
Where, moment of inertia of a rod would be,
We could equate (2) and (3) as the system is conservative,
Therefore, we found the angular velocity to be,
Hence, option B is correct.
Note: Now, you may be wondering why haven’t we discussed anything about the translational kinetic energy. This is simply because it just doesn’t exist here. You may find that the point at which the rod is hinged doesn’t move and is fixed and thereby we shouldn’t worry about the translational motion and also the related kinetic energy.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




