
A rod of length
(A)
(B)
(C)
(D) The relation between
Answer
447.9k+ views
Hint :Here, we have to use the concept of rotational motion. As we have been given the rod of some length and asked to tie its one end to the fixed point and observe the tensions acting at some distances away from pivoted ends. Here, tension is equal to the centrifugal force acting on the end of the body which is rotating.
Complete Step By Step Answer:
We have the rod of length
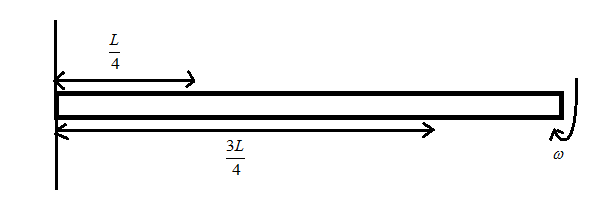
Let us first consider the part of length of
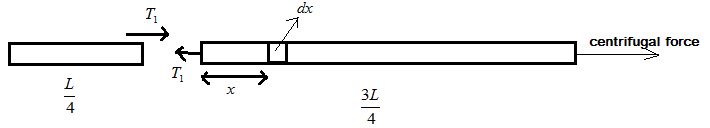
Here, we have resolved all the forces that are responsible for the tension in the rod, also let
Thus, mass per unit length,
Now tension acting on length
Therefore,
Now, we know that the
Such that,
Similarly, for
We have to find tension
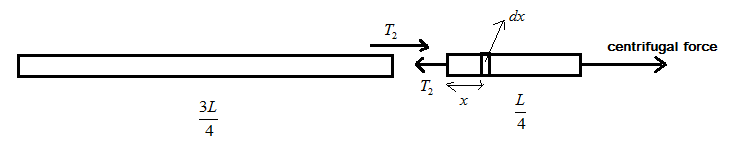
Follow the same procedure with limits
Thus, from above conclusions we get that the tensions
The correct answer is option A.
Note :
In these types of problems it is important to resolve the forces acting on each other partly or wholly because in the question the tension is asked at different lengths. We carried out the expressions by equating the forces and observed the answer and found out the comparison between tensions. This question can also be solved with the help of the concept of MOI of the rod. You can try yourself.
Complete Step By Step Answer:
We have the rod of length
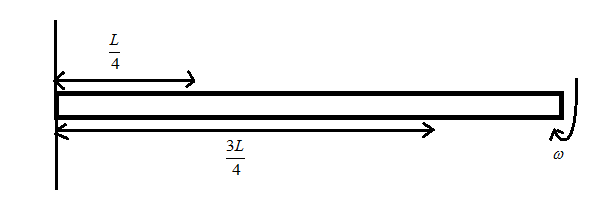
Let us first consider the part of length of
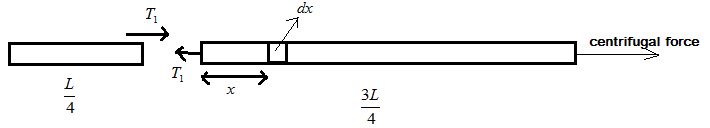
Here, we have resolved all the forces that are responsible for the tension in the rod, also let
Thus, mass per unit length,
Now tension acting on length
Therefore,
Now, we know that the
Such that,
Similarly, for
We have to find tension

Follow the same procedure with limits
Thus, from above conclusions we get that the tensions
The correct answer is option A.
Note :
In these types of problems it is important to resolve the forces acting on each other partly or wholly because in the question the tension is asked at different lengths. We carried out the expressions by equating the forces and observed the answer and found out the comparison between tensions. This question can also be solved with the help of the concept of MOI of the rod. You can try yourself.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




