
A sector containing an angle of
Answer
512.7k+ views
Hint: First of all, we will find the radius of base of the cone and then, we will find the slant height of the cone and we will use the formula of curved surface area of cone given by as follows,
Where, r is radius and l is slant height of a cone.
Also, we will use the formula to find the length of the arc given by
Complete step-by-step answer:
We have been given a sector containing an angle of
Let the base radius of the cone be r and l be the slant height.
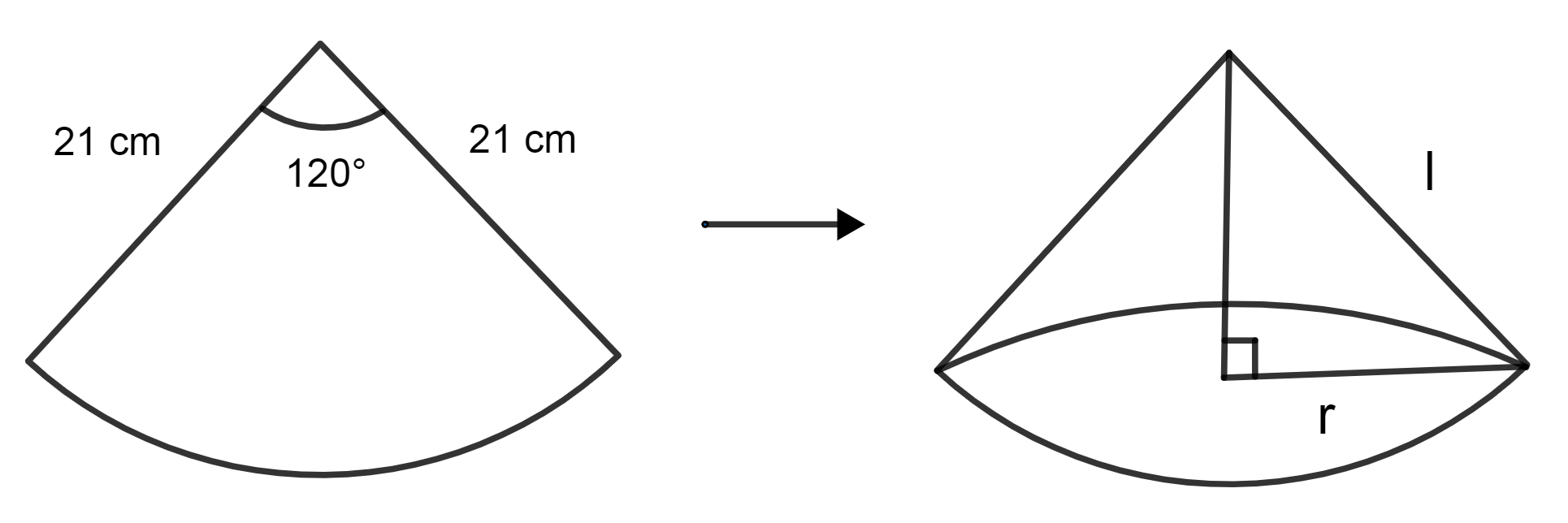
Since, the sector is folded into a right circular cone, we have circumference of the base of the cone = length of the arc.
We know that, length of an arc having radius R which subtend an angle
Cancelling similar terms on both sides, we get:
Now, we can substitute
Thus, the base radius of cone = 7cm.
Also, the slant height (l) of the cone = Radius of the sector.
Thus, l = R = 21cm.
Now, we know that,
Thus, the curved surface area of the cone is 462 sq.cm
Note: We can also solve this question in less time, if we know that the area of the sector is equal to the area of cone formed by the sector. So, we will find the area of sector using the formula
Where, r is radius and l is slant height of a cone.
Also, we will use the formula to find the length of the arc given by
Complete step-by-step answer:
We have been given a sector containing an angle of
Let the base radius of the cone be r and l be the slant height.

Since, the sector is folded into a right circular cone, we have circumference of the base of the cone = length of the arc.
We know that, length of an arc having radius R which subtend an angle
Cancelling similar terms on both sides, we get:
Now, we can substitute
Thus, the base radius of cone = 7cm.
Also, the slant height (l) of the cone = Radius of the sector.
Thus, l = R = 21cm.
Now, we know that,
Thus, the curved surface area of the cone is 462 sq.cm
Note: We can also solve this question in less time, if we know that the area of the sector is equal to the area of cone formed by the sector. So, we will find the area of sector using the formula
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




