
A ship A is moving Westwards with a speed of
Answer
487.8k+ views
2 likes
Hint: Here, the velocities of ships A and B are given. From this, we can find the velocity of A with respect to B. Then, draw a vector diagram and mark the distances and angles. Consider the triangle made by the resultant velocity and the path of ship B and find out all the sides and thereby the time taken by the ships to reach their shortest distance can be calculated.
Formula used:
Complete step by step answer:
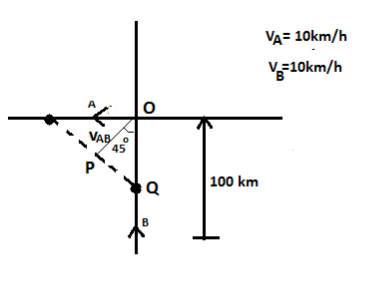
Velocity of A with respect to B is,
Then,
Given that,
Velocity of A,
Velocity of B,
Substitute the velocities of ships A and B in equation 1 we get,
Velocity of A with respect to B,
From the above diagram the shortest distance between ships A and B is PQ.
Consider triangle POQ,
Then,
We have,
Substitute the values of PQ and
Then, the time taken to reach the shortest distance,
Therefore, the answer is option B.
Note:
Alternate method to solve the question
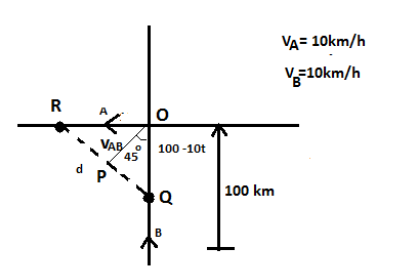
Given,
Velocity of A,
Velocity of B,
Given that, both ships A and B are travelling with the same velocity. Then, at any instant
i.e.,
Distance travelled by ship A = Distance travelled by ship B
Then, the remaining distance for B is,
Then, considering triangle ROQ,
Differentiating both sides with respect to time,
Formula used:
Complete step by step answer:

Velocity of A with respect to B is,
Then,
Given that,
Velocity of A,
Velocity of B,
Substitute the velocities of ships A and B in equation 1 we get,
Velocity of A with respect to B,
From the above diagram the shortest distance between ships A and B is PQ.
Consider triangle POQ,
Then,
We have,
Substitute the values of PQ and
Then, the time taken to reach the shortest distance,
Therefore, the answer is option B.
Note:
Alternate method to solve the question

Given,
Velocity of A,
Velocity of B,
Given that, both ships A and B are travelling with the same velocity. Then, at any instant
i.e.,
Distance travelled by ship A = Distance travelled by ship B
Then, the remaining distance for B is,
Then, considering triangle ROQ,
Differentiating both sides with respect to time,
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




