
A sinusoidal wave propagates along a string. In figure (a) and (b). ‘y’ represents displacement of particles from the mean position. ‘x’ and ‘t’ have usual meanings. Find: maximum velocity and maximum acceleration of the particles.



Answer
480.3k+ views
Hint: Velocity is defined as the rate of change of displacement of a particle. And the acceleration of a particle is the rate of change of velocity of that particle. Calculate angular acceleration ‘w’ and maximum amplitude ‘A’ using their mathematical expressions. Then find out the values of maximum velocity and maximum acceleration of particles by deducing the linear equivalent of these rotational quantities i.e. angular acceleration and angular velocity.
Formula used:
Complete answer:
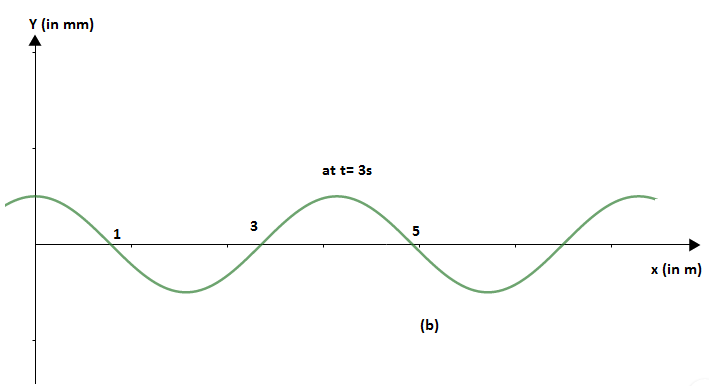
We must study the given figures carefully first. After careful consideration we can notice that from both figures (a) and (b) the values of t = 4sec. Now, we need to find the value of angular acceleration. Using the mathematical expression for angular velocity we have:
Amplitude is A= 3mm
Let’s find out the maximum velocity and acceleration of the particle we know that
Where, ‘ω’ represents the angular velocity and ‘A’ represents the amplitude
Now acceleration of the particle can be found by using the following formula.
Therefore, maximum velocity and maximum acceleration of the particles are
So, the correct answer is “Option A”.
Note:
It is advised to clearly understand the figures given in the question and then solve the further question. We need to remember the units of ω that are rad/sec. Additionally, notice how the angular velocity and angular acceleration are related to their linear quantities. These are useful expressions. Similarly, every rotational quantity can be converted into its linear equivalent by multiplying it with its proportionality constant with the linear quantity.
Formula used:
Complete answer:
We must study the given figures carefully first. After careful consideration we can notice that from both figures (a) and (b) the values of t = 4sec. Now, we need to find the value of angular acceleration. Using the mathematical expression for angular velocity we have:
Amplitude is A= 3mm
Let’s find out the maximum velocity and acceleration of the particle we know that
Where, ‘ω’ represents the angular velocity and ‘A’ represents the amplitude
Now acceleration of the particle can be found by using the following formula.
Therefore, maximum velocity and maximum acceleration of the particles are
So, the correct answer is “Option A”.
Note:
It is advised to clearly understand the figures given in the question and then solve the further question. We need to remember the units of ω that are rad/sec. Additionally, notice how the angular velocity and angular acceleration are related to their linear quantities. These are useful expressions. Similarly, every rotational quantity can be converted into its linear equivalent by multiplying it with its proportionality constant with the linear quantity.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




