
A small body of mass m tied to a non-stretchable thread moves over a smooth horizontal plane. The other end of the thread is being drawn into a hole
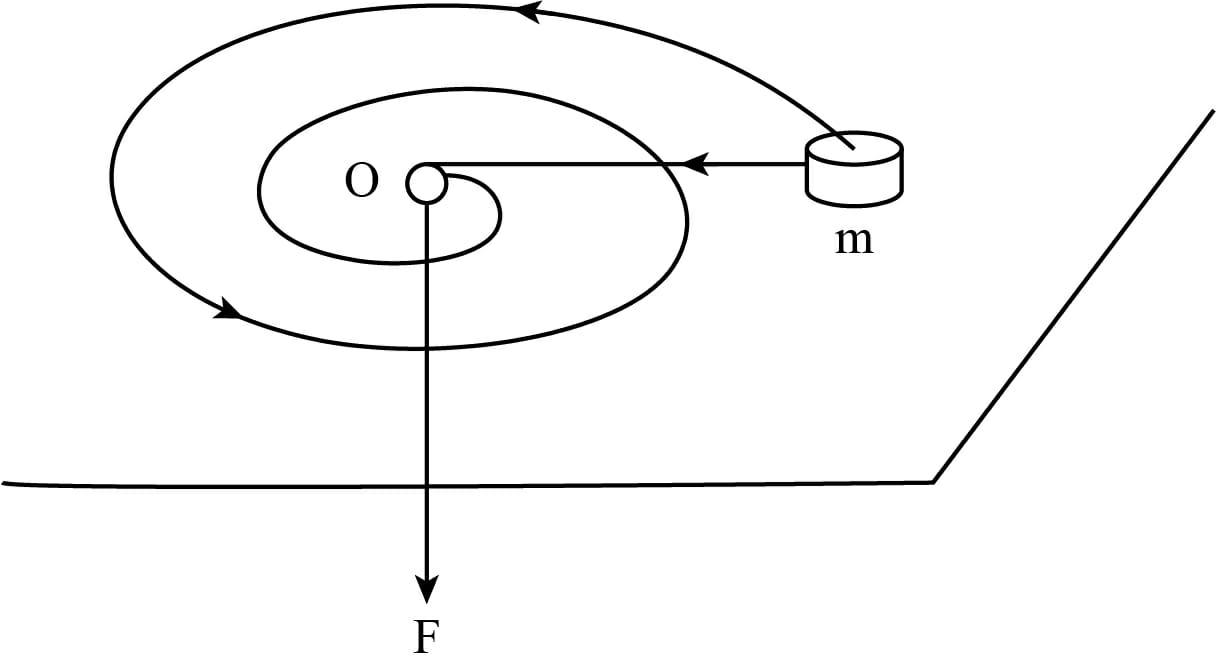

Answer
506.1k+ views
Hint: To solve this problem we use the concept of conservation of angular momentum since the momentum of force
Complete step by step answer:
Forces, acting on the mass m are shown in the figure. As a vector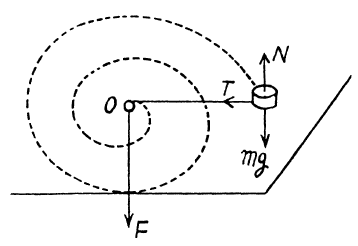
Let, the angular velocity of the particle be
Also
Now from the Newton’s second law of motion,
Note:
Law of conservation of angular momentum states that if no external torque acts on the object, then there is no angular momentum.
Because the momentum of
So, the angular momentum is conserved.
Complete step by step answer:
Forces, acting on the mass m are shown in the figure. As a vector

Let, the angular velocity of the particle be
Also
Now from the Newton’s second law of motion,
Note:
Law of conservation of angular momentum states that if no external torque acts on the object, then there is no angular momentum.
Because the momentum of
So, the angular momentum is conserved.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




