
A smooth inclined plane having angle of inclination
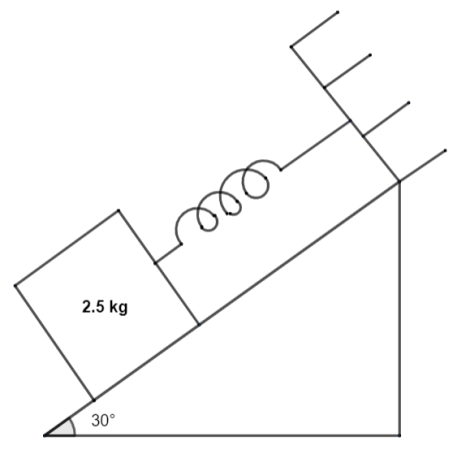

Answer
500.1k+ views
Hint: We will use the formula of angular velocity as the surface is of the inclined plane. It is clear from the question that there is no tension on the spring so, by the use of the direct formula of induced force equal to force of inclination will be useful for angular frequency.
Formula used:
Complete answer:
Angular frequency: By the term angular frequency we mean the number of events that are occurring per unit time.
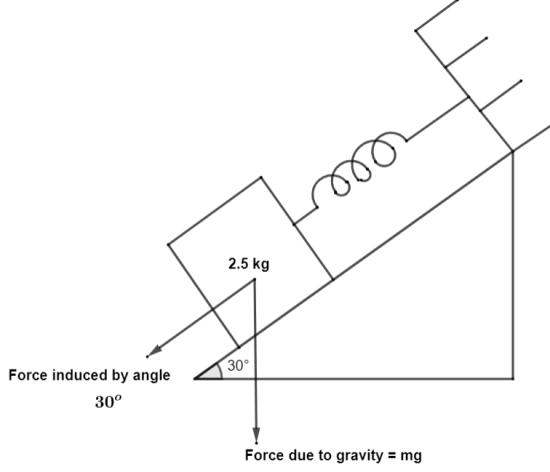
As the weight attached to the spring with a mass of 2.5 kg does create tension in the spring. But in the question it is given that the tension is reduced to zero. This results in the fact that the string is not at all stretched. As the force created is with an angle
This force will be further equal to the spring constant, the product of the spring constant and the extension of the spring. Therefore,
Since, the mass of the object is 2.5 cm or 0.025 m upwards but along the surface of the inclined plane, so the extension of spring due to the mass x = 0.025 m.
As,
Thus,
Since, the force is at some angle so, there will be an angular velocity
By the SI unit of angular velocity to be
Hence, the correct option is
So, the correct answer is “Option D”.
Note:
To solve such type of questions one should remember the following points:
(1) There can be confusion between the values of the spring constant K and its extension by the mass so, for this we will check the units here. Since, the unit of mass is used as kg and extension has used cm as a unit so, the confusion will get resolved.
(2) The concept of angular frequency must be on fingertips.
(3) Always remember the SI unit of angular velocity as
Formula used:
Complete answer:
Angular frequency: By the term angular frequency we mean the number of events that are occurring per unit time.

As the weight attached to the spring with a mass of 2.5 kg does create tension in the spring. But in the question it is given that the tension is reduced to zero. This results in the fact that the string is not at all stretched. As the force created is with an angle
This force will be further equal to the spring constant, the product of the spring constant and the extension of the spring. Therefore,
Since, the mass of the object is 2.5 cm or 0.025 m upwards but along the surface of the inclined plane, so the extension of spring due to the mass x = 0.025 m.
As,
Thus,
Since, the force is at some angle so, there will be an angular velocity
By the SI unit of angular velocity to be
Hence, the correct option is
So, the correct answer is “Option D”.
Note:
To solve such type of questions one should remember the following points:
(1) There can be confusion between the values of the spring constant K and its extension by the mass so, for this we will check the units here. Since, the unit of mass is used as kg and extension has used cm as a unit so, the confusion will get resolved.
(2) The concept of angular frequency must be on fingertips.
(3) Always remember the SI unit of angular velocity as
Latest Vedantu courses for you
Grade 6 | CBSE | SCHOOL | English
Vedantu 6 Pro Course (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




