Question

When a solid block is fully immersed in water, the volume of the water displaced is:
A) greater than the volume of the block.
B) less than the volume of the block.
C) Equal to the volume of the block.
D) Depends upon the manner in which the block is immersed in the liquid.

Answer 1

Hint: Here a block is fully immersed in water and some of the volume is displaced. We have to tell the amount of volume displaced in comparison to the volume of the block. And we know about Archimedes’ Principle which tells us the relation between the weight of the body which is immersed in liquid and the weight of liquid displaced, hence by using this principle we will find the answer.

Formula used:
\[\dfrac{\text{density of object}}{\text{density of fluid}}\text{ = }\dfrac{\text{weight of object}}{\text{weight of displaced fluid}}\]

Complete answer:
According to Archimedes’ principle an object immersed in fluid experiences an upthrust force or buoyant force due to which it floats. This force is given as the product of density of fluid, volume of liquid displaced and acceleration due to gravity.

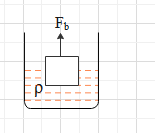
\[{{F}_{b}}=\rho Vg\]
Here g is acceleration due to gravity, V is volume of fluid displaced and ρ is the density of the fluid.
Now according to Archimedes’ principle the volume of the fluid displaced is directly proportional to the volume of the block and for an object which is fully immersed the volume of displaced fluid will be equal to volume of the object. A simple diagram can be given as

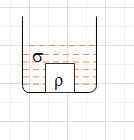
This can be proved by the relation between the density of object, its weight and density of fluid and weight of displaced fluid is given as
\[\dfrac{\text{density of object}}{\text{density of fluid}}\text{ = }\dfrac{\text{weight of object}}{\text{weight of displaced fluid}}\]
We can rewrite the above equation as
\[\text{density of object}\times \text{weight of displaced fluid}=\text{density of fluid}\times \text{weight of object}\]
Now we know that weight is actually force which is experienced due to gravitational pull and it is given as a product of mass and acceleration due to gravity. If mass of object is m, mass of displaced fluid is m’ and density of object is ρ and density of fluid is σ, then we can rewrite the above equation as
\[\rho \times m'g=\sigma \times mg\text{ }.......\text{(i)}\]
Now density of an object is given as mass per unit volume i.e.
\[\begin{align}
  & \rho =\dfrac{m}{V} \\
 & \Rightarrow m=\rho V \\
\end{align}\]
 As we want the volume term so we can substitute value of mass as product of density and volume. If V is the volume of object and V’ is volume of displaced fluid then substituting value of m and m’ as product of density and volume, we get
\[\rho \times \left( \sigma V' \right)g=\sigma \times \left( \rho V \right)g\]
Here, the density of displaced fluid will be the same as the density of fluid because it is basically mass per unit volume which will not change with change in quantity. Now cancelling the common terms on both sides we get,
\[V'=V\]
Which means the volume of displaced fluid will be the same as the volume of the object when immersed totally.

So, the correct answer is “Option C”.

Note:
Most of the time we get confused in mass and weight and consider both the same. But in reality these are two different quantities, when we say weight, it means it gives us the amount of force which is acted on a body due to its mass. We can say it is the gravitational force. Even the unit of weight is Newton and not kilogram which is a unit of mass.