
A solid cylinder rolls down an incline plane. Its mass is 2 kg and radius 0.1 m. If the height of the inclined plane is 4 m, its rotational kinetic energy, when it reaches the foot of the plane is:
(A) 78.4 J
(B) 39.2 J
(C)
(D) 19.6 J
Answer
441.3k+ views
1 likes
Hint: The sum of translational and rotational kinetic energy of the cylinder will give the total kinetic energy of the cylinder at the bottom of the incline. This energy is taken from the potential energy that it stored in the cylinder at a height of 4 m.
Formula used:
The loss in potential energy turns into to the gain in kinetic energy at the bottom of the incline:
Loss in P.E. = Gain in K.E.
In rotational motion, the angular velocity of motion is written as:
Rotational kinetic energy is given as:
Complete answer:
Let the mass of the cylinder be denoted by m, the radius be denoted by r and the moment of inertia be denoted by I.
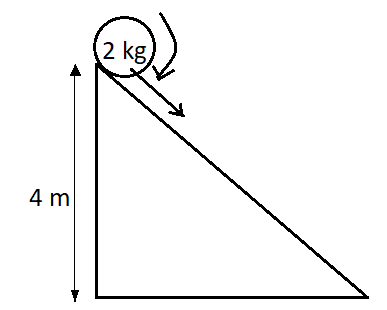
The total kinetic energy for the cylinder is written as:
We know that for the case of cylinder the moment of inertia is written as:
Substituting this in the kinetic energy expression, we get:
Now, we also substitute the frequency of rotational motion of the cylinder here:
The potential energy at the top of h height is written as:
P.E. = mgh.
Equating this with the obtained potential energy we get:
The velocity is obtained from this expression as:
The rotational kinetic energy is:
where substitution for I and angular velocity has been made.
Keeping the value of v in this expression, we get rotational kinetic energy as:
Note:
The value of g has been kept as 9.8 instead of 10 m/s
Formula used:
The loss in potential energy turns into to the gain in kinetic energy at the bottom of the incline:
Loss in P.E. = Gain in K.E.
In rotational motion, the angular velocity of motion is written as:
Rotational kinetic energy is given as:
Complete answer:
Let the mass of the cylinder be denoted by m, the radius be denoted by r and the moment of inertia be denoted by I.

The total kinetic energy for the cylinder is written as:
We know that for the case of cylinder the moment of inertia is written as:
Substituting this in the kinetic energy expression, we get:
Now, we also substitute the frequency of rotational motion of the cylinder here:
The potential energy at the top of h height is written as:
P.E. = mgh.
Equating this with the obtained potential energy we get:
The velocity is obtained from this expression as:
The rotational kinetic energy is:
where substitution for I and angular velocity has been made.
Keeping the value of v in this expression, we get rotational kinetic energy as:
Note:
The value of g has been kept as 9.8 instead of 10 m/s
Latest Vedantu courses for you
Grade 10 | ICSE | SCHOOL | English
Vedantu ICSE 10 Pro Course (2025-26)
School Full course for ICSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




