Question

A source is producing 15 waves in 3 seconds. The distance between a crest and the adjacent trough is 10 cm. Find the velocity of the wave?
A. 1cm/s
B. 1.5cm/s
C. 1m/s
D. 2m/s

Answer 1

Hint: From the question you can find the number of waves produced per unit second and hence the frequency of the wave. Also, recall that the distance between adjacent crests and trough is half the wavelength and hence find the wavelength. Now we can directly substitute these values in the expression for velocity and hence find the answer.

Formula used:
Expression for velocity in terms of wavelength and frequency,
 $ v=f\lambda $

Complete step-by-step answer:
We are given a source that is producing 15 waves every 3 seconds. We are also given the distance between the adjacent crest and trough as 10cm and then we are asked to find the velocity of the wave.
We know that the frequency of a wave is defined as the number of waves produced per unit second. It is also given by the reciprocal of the time period. The SI unit of frequency is Hertz (Hz) or $ \left( {{s}^{-1}} \right) $ .
In the question, the source is producing 15 waves every 3 seconds, that is,
 $ \Rightarrow 3 $ -seconds $ \to 15 $ waves
 $ \Rightarrow 1s\to \dfrac{15}{3} $ -waves
 $ \Rightarrow 1s\to $ 5 waves
That is every unit second the source is producing 5 waves, that is, the frequency of the wave is, 5Hz.
 $ \Rightarrow f=5Hz $ …………………………… (1)

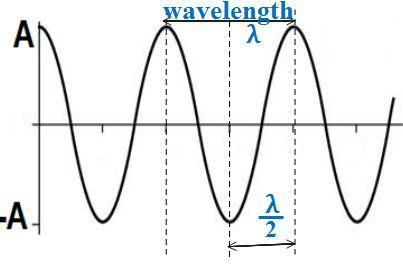
We are given the distance between adjacent crests and trough as 10cm. From the above figure it is quite clear that the distance between adjacent crests and trough is half the wavelength of the wave. Therefore,
 $ \dfrac{\lambda }{2}=10cm $
 $ \Rightarrow \lambda =20cm $ …………………………. (2)
So we get the wavelength of the given wave as 20 cm.
Now that we got the required quantities to solve the problem, let us find the velocity of the given wave. For that, let us recall the relation connecting the frequency, wavelength and velocity which is given by,
 $ f=\dfrac{v}{\lambda } $
 Where, f is the frequency, v is the velocity and λ is the wavelength of the wave. Substituting (1) and (2), we get,
 $ \Rightarrow v=f\lambda =5\times 20=100cm{{s}^{-1}} $
 $ \Rightarrow v=1m{{s}^{-1}} $
Therefore, we get the velocity of the given wave as $ 1m{{s}^{-1}} $ .

So, the correct answer is “Option C”.

Note: All the required quantities are actually indirectly given in the question itself. By definition of the wavelength it is the distance between adjacent crests or adjacent troughs then obviously the distance between the adjacent crest and trough should be the value of half wavelength. Also, convert the final answer according to that given in the options.