
A square has two of its vertices on a circle and other two on a triangle to the circle. If the diameter of the circle is 10cm, then the side of the square is?
Answer
483.9k+ views
1 likes
Hint: In this question, we will first draw a diagram for better understanding. After that, we will suppose the side of the square as x. Using the following properties, we will evaluate our answer.
(i) Radius is the half of diameter i.e.
(ii) Pythagoras theorem:
Complete step by step answer:
According to our question, the square has two vertices on a circle and two vertices on a tangent to the circle. Let us assume that the square ACFE whose vertices A and C touch the circle and E and F touches the tangent to the circle at point of contact D. Let O be the center of the circle. Join OA, OD and OC. Our diagram looks like this,
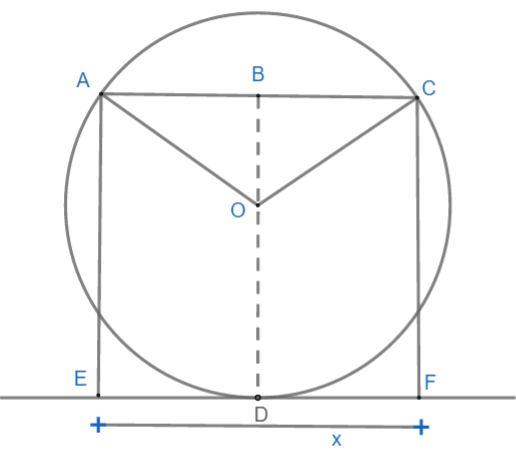
Let BD be a line in the center of the square such that it bisects AC and passes through the center.
This line BD will be perpendicular to AC (because AE and CF are perpendicular to AC as it is a square).
Now let us suppose that the side of the square is x cm.
As we are given that, the diameter of the circle is 10cm, therefore, the radius of the circle will be half of the diameter. Hence radius
From the diagram we can see that OA, OC and OD are the radius of the circle.
So, OA = 5cm, OC = 5cm and OD = 5cm.
Since the side of the square is x cm, so AC = x cm.
Half of AC is AB, so
Since BD is equal to CF, which is the side of square. So BD = x cm.
Now, BD = OB + OD but BD = x cm and OD = 5cm, so we get,
Hence in
According to Pythagoras theorem,
In
Applying
Taking LCM as 4 we get,
Taking 4 to the other side we get,
This means either x = 0 or (5x-40)=0.
Since the side of the square cannot be 0. So,
Dividing by 5 on both sides we get,
Since x was supposed to be the side of the square therefore, side of the square is 8cm.
Note: Students should note that diagrams are must for this question, otherwise students can easily make mistakes. For the proof of OB being the perpendicular bisector of AC we can use following proof: since OA and OC are equal so
(i) Radius is the half of diameter i.e.
(ii) Pythagoras theorem:
Complete step by step answer:
According to our question, the square has two vertices on a circle and two vertices on a tangent to the circle. Let us assume that the square ACFE whose vertices A and C touch the circle and E and F touches the tangent to the circle at point of contact D. Let O be the center of the circle. Join OA, OD and OC. Our diagram looks like this,

Let BD be a line in the center of the square such that it bisects AC and passes through the center.
This line BD will be perpendicular to AC (because AE and CF are perpendicular to AC as it is a square).
Now let us suppose that the side of the square is x cm.
As we are given that, the diameter of the circle is 10cm, therefore, the radius of the circle will be half of the diameter. Hence radius
From the diagram we can see that OA, OC and OD are the radius of the circle.
So, OA = 5cm, OC = 5cm and OD = 5cm.
Since the side of the square is x cm, so AC = x cm.
Half of AC is AB, so
Since BD is equal to CF, which is the side of square. So BD = x cm.
Now, BD = OB + OD but BD = x cm and OD = 5cm, so we get,
Hence in
According to Pythagoras theorem,
In
Applying
Taking LCM as 4 we get,
Taking 4 to the other side we get,
This means either x = 0 or (5x-40)=0.
Since the side of the square cannot be 0. So,
Dividing by 5 on both sides we get,
Since x was supposed to be the side of the square therefore, side of the square is 8cm.
Note: Students should note that diagrams are must for this question, otherwise students can easily make mistakes. For the proof of OB being the perpendicular bisector of AC we can use following proof: since OA and OC are equal so
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




