
A stone is projected horizontally with a speed v from a height h above the ground. A horizontal wind is blowing in direction opposite to velocity of projection and gives the stone a constant horizontal acceleration of (direction opposite to initial velocity). As a result, the stone falls on ground at point vertically below the point of projection. Then find the value of
Answer
497.4k+ views
Hint: We need to consider the equations of motion of an object in two-dimensions to solve this problem. We can find relations between the given parameters and relate them together to reach the desired solution for the given expression.
Complete step by step answer:
In a projectile motion, an object is fired with a velocity that has its components along both the vertical and horizontal directions. In the situation given to us, the stone is projected horizontal, i.e., there is no vertical force and therefore, a vertical velocity or acceleration during the time of flight.
In a 2-D motion, the equation of motion can be given as –
Where, S is the displacement along an axis,
u is the initial velocity,
g is the acceleration due to gravity,
t is the time of flight.
Let us consider our situation now. It is given that the stone has a horizontal velocity v at
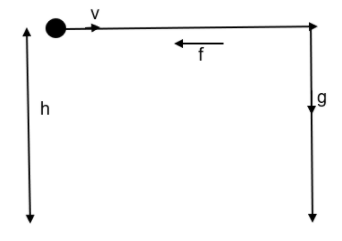
Due to the opposite horizontal force (acceleration f) the stone reaches an abrupt stop and falls vertically downwards under the force of gravity at an acceleration of g.
Applying the equation of motion, we get,
Where h is the vertical height. The initial velocity is 0 at the start of vertical motion.
Also, at this point there is no more horizontal displacement.
The equation of motion for the horizontal motion becomes,
Also, we have another equation for time of flight as in (1), So equating (1) and (2),
So, the required answer is 2.
Note:
We can easily use this time of flight equation in 1-D for this question because here involves no parabolic motion after the horizontal flight. The stone tends to move down vertically at the instant when its initial velocity matches with the opposing velocity due to wind force.
The vertical motion is due to the cancelling of horizontal velocities.
Complete step by step answer:
In a projectile motion, an object is fired with a velocity that has its components along both the vertical and horizontal directions. In the situation given to us, the stone is projected horizontal, i.e., there is no vertical force and therefore, a vertical velocity or acceleration during the time of flight.
In a 2-D motion, the equation of motion can be given as –
Where, S is the displacement along an axis,
u is the initial velocity,
g is the acceleration due to gravity,
t is the time of flight.
Let us consider our situation now. It is given that the stone has a horizontal velocity v at

Due to the opposite horizontal force (acceleration f) the stone reaches an abrupt stop and falls vertically downwards under the force of gravity at an acceleration of g.
Applying the equation of motion, we get,
Where h is the vertical height. The initial velocity is 0 at the start of vertical motion.
Also, at this point there is no more horizontal displacement.
The equation of motion for the horizontal motion becomes,
Also, we have another equation for time of flight as in (1), So equating (1) and (2),
So, the required answer is 2.
Note:
We can easily use this time of flight equation in 1-D for this question because here involves no parabolic motion after the horizontal flight. The stone tends to move down vertically at the instant when its initial velocity matches with the opposing velocity due to wind force.
The vertical motion is due to the cancelling of horizontal velocities.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




