
A student is experimenting with a resonance tube apparatus in a Physics lab to find the speed of sound at room temperature. He got resonating lengths of air columns as
A.
B.
C.
D.
Answer
495k+ views
Hint: The resonance tube is considered to be an open organ pipe. So, for first resonance, there is one node and one antinode, whose length is a quarter of the wavelength of the sound wave. The water inside the reservoir will move upward, as the resonances increase, successively.
Formula used:
Complete answer:
First let us draw a rough diagram of resonance tube apparatus. It is mentioned in the problem that the student got resonating columns at
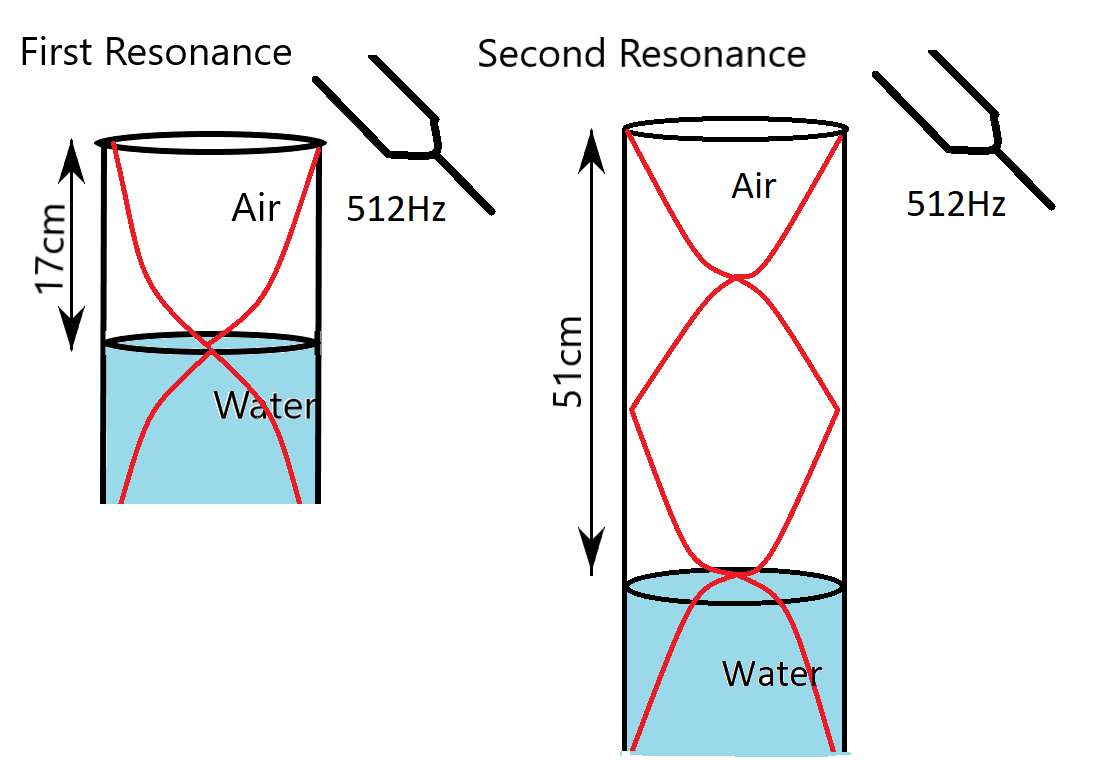
The condition for first resonance is
Where
We have
Now, for the second part of the answer, we have actually increased the air column compared to the first resonance. So, the water level would have reduced, in the resonance tube. This will compensate as an increase of water level in the side water reservoir. This shows as an upward movement of water in the side water reservoir.
So, the correct answer is “Option B”.
Note:
The above problem can also be solved using the condition for second resonance i.e.,
Where
Substituting the values given, we have
Formula used:
Complete answer:
First let us draw a rough diagram of resonance tube apparatus. It is mentioned in the problem that the student got resonating columns at

The condition for first resonance is
Where
We have
Now, for the second part of the answer, we have actually increased the air column compared to the first resonance. So, the water level would have reduced, in the resonance tube. This will compensate as an increase of water level in the side water reservoir. This shows as an upward movement of water in the side water reservoir.
So, the correct answer is “Option B”.
Note:
The above problem can also be solved using the condition for second resonance i.e.,
Where
Substituting the values given, we have
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




