
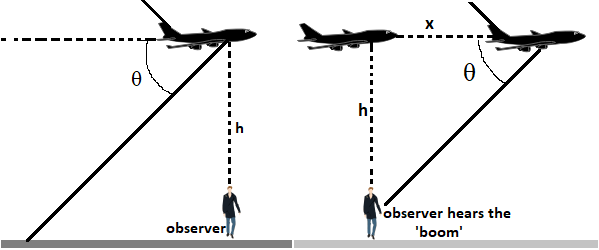
A supersonic jet travelling at Mach 3.00 at an altitude of 20000 m is directly over a person at time t = 0 as shown in Figure.
How long will it be before the person encounters the shock wave?

Answer
494.4k+ views
Hint: In the question there is a jet travelling at mach 3.00. a person is standing directly under the jet. The height from the observer to the jet is given to us. We need to find the time taken for the shock wave to reach the observer. We know the equation to find the shock angle and from the figure we can find the change in position of the jet. Using this we can derive for velocity and thus get the time taken.
Formula used:
Shock angle,
Velocity,
Complete answer:
In the question it is said that a supersonic jet is travelling at an altitude ‘h’ and h is given to us.
h = 20000
The mach number of the jet is given to us as 3.00
We know that the average speed of sound in air is 335 m/s.
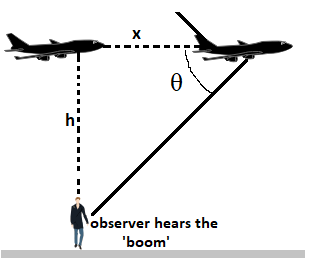
In the figure the angle ‘
We know the equation for the shock angle,
We know that velocity of mach is the product of Mach number and velocity of sound in air, i.e.
Therefore we get,
From this we get the shock angle as,
From the figure we get tan of the angle ‘
We know the value of ‘
This is the change in position of the jet.
We need to find the taken by the observer to hear the sound.
We now know that velocity is the derivative of position with respect to time.
Therefore here we have,
In this above equation we know the values of
This is the time taken by the observer to hear the shock wave.
Note:
When a wave is propagating and the medium of propagation does not change, then there is no change in the velocity of the sound wave. But if there is a relative motion between the source and the observer, then the frequency of the wave will change accordingly. This phenomenon is called the Doppler Effect in sound.
While solving the question we take velocity as the derivative of position with respect to time. In this case the change in position is the value marked as ‘x’ in the figure.
Formula used:
Shock angle,
Velocity,
Complete answer:
In the question it is said that a supersonic jet is travelling at an altitude ‘h’ and h is given to us.
h = 20000
The mach number of the jet is given to us as 3.00
We know that the average speed of sound in air is 335 m/s.

In the figure the angle ‘
We know the equation for the shock angle,
We know that velocity of mach is the product of Mach number and velocity of sound in air, i.e.
Therefore we get,
From this we get the shock angle as,
From the figure we get tan of the angle ‘
We know the value of ‘
This is the change in position of the jet.
We need to find the taken by the observer to hear the sound.
We now know that velocity is the derivative of position with respect to time.
Therefore here we have,
In this above equation we know the values of
This is the time taken by the observer to hear the shock wave.
Note:
When a wave is propagating and the medium of propagation does not change, then there is no change in the velocity of the sound wave. But if there is a relative motion between the source and the observer, then the frequency of the wave will change accordingly. This phenomenon is called the Doppler Effect in sound.
While solving the question we take velocity as the derivative of position with respect to time. In this case the change in position is the value marked as ‘x’ in the figure.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




