Question

A thin biconvex lens of refractive index $\dfrac{3}{2}$ is placed on a horizontal plane mirror as shown. The space between the lens and the mirror is then filled with water of refractive index $\dfrac{4}{3}$ . It is found that when a point object is placed $15\,cm$ above the lens on its principal axis, the object coincides with its own images. On representing another liquid, the object and the image again coincide at a distance $25cm$ from the lens. Calculate the refractive index of the liquid.

Answer 1

Hint:Here we will solve this question in two cases in which the first one will be When the space between the lens and mirror is filled by water of refractive index ${\mu _1}$ and in second one where the refractive index will be ${\mu _2}$ . The light retraced its path if it is incident normally on a mirror. The ray after refraction through the lens and the liquid are parallel. We will apply the general thin lens equation with parameters.

Formula used:
$\dfrac{1}{f} = (n - 1)\left( {\dfrac{1}{{{R_1}}} - \dfrac{1}{{{R_2}}}} \right)$
Where, $n$ is the refractive index, $R_1$ and $R_2$ are the radii of curvature.,$R_1$ is denoted as the surface very near to the light source and $R_2$ is denoted as the surface very far from the light source.

Complete step by step answer:

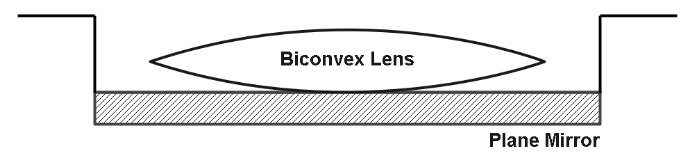
According to the question, let ${f_1}$​ be the focal length of a convex lens; radius of curvature of each curved face is $R$.
$\dfrac{1}{{{f_1}}} = (m - 1)\left( {\dfrac{1}{R} - \left( {\dfrac{1}{{ - R}}} \right)} \right) \\
\Rightarrow \dfrac{1}{{{f_1}}}= (\mu - 1)\dfrac{2}{R} \\
\Rightarrow {f_1} = \dfrac{R}{{2(\mu - 1)}} \\
\Rightarrow {f_1}= \dfrac{R}{{2\left( {\dfrac{3}{2} - 1} \right)}} \\
\Rightarrow {f_1}= R \\ $
When the space between the lens and mirror is filled by water of refractive index ${\mu _1} = \dfrac{4}{3}$ , then the focal length of liquid concave lens ${f_2}$​ is
$\dfrac{1}{{{f_2}}} = ({\mu _1} - 1)\left( { - \dfrac{1}{R} - \infty } \right) \\
\Rightarrow {f_2} = \dfrac{{ - R}}{{{\mu _1} - 1}} \\
\Rightarrow {f_2}= - \dfrac{R}{{\left( {\dfrac{4}{3} - 1} \right)}} \\
\Rightarrow {f_2}= - 3R \\ $
The combined focal length of lenses is ${F_1} = 15cm$
$\therefore \dfrac{1}{{{F_1}}} = \dfrac{1}{{{f_1}}} + \dfrac{1}{{{f_2}}}$
Which is given by, if two lenses are considered as equivalent to a single lens of focal length f,
$\dfrac{1}{{15}} = \dfrac{1}{R} - \dfrac{1}{{3R}} \\
\Rightarrow \dfrac{1}{{15}} = \dfrac{{3 - 1}}{{3R}} \\
\Rightarrow 3R = 30 \\
\Rightarrow R = 10cm \\ $
In the second case,
${F_2} = 25\,cm$
Let, ${\mu _1} = {\mu _2}$
Similarly, we will apply same as previous
If two lenses are considered as equivalent to a single lens of focal length f, is given by
$\dfrac{1}{{{F_2}}} = \dfrac{1}{{{f_1}}} + \dfrac{1}{{{f_2}}}$
Now, equating, we will get ${f'_2}$ .
$\dfrac{1}{{25}} = \dfrac{1}{{10}} + \dfrac{1}{{{{f'}_2}}} \\
\Rightarrow \dfrac{1}{{{{f'}_2}}} = \dfrac{1}{{25}} - \dfrac{1}{{10}} \\
\Rightarrow \dfrac{1}{{{{f'}_2}}} = \dfrac{{2 - 5}}{{50}} \\
\Rightarrow {{f'}_2} = \dfrac{{ - 50}}{3}cm \\ $
Now, finally we have to calculate the refractive index of the liquid,
${{f'}_2} = \dfrac{R}{{{\mu _2} - 1}} \\
\Rightarrow {\mu _2} - 1 = - \dfrac{R}{{{{f'}_2}}} \\
\Rightarrow {\mu _2} - 1 = \dfrac{{ - 10}}{{\left( { - \dfrac{{50}}{3}} \right)}} \\ $
Now, cross multiplying,
${\mu _2} - 1 = \dfrac{3}{5} = 0.6 \\
\Rightarrow {\mu _2} = 1 + 0.6 \\
\therefore {\mu _2} = 1.6 \\ $
Hence, the refractive index of the liquid is $1.6$.

Note:Don’t get confused on the refractive indexes, solve all the equations step wise to avoid mistakes. Remember the formula and we know that, generally, a convex lens can converge a beam of parallel rays to a point on the other side of the lens. This point is called a focus of the lens and its distance from the Optical Center of the beam is called the focal length. The radius of curvatures $R_1$ and $R_2$ of the spherical surfaces and the focal length of the lens ‘f’ are connected by an approximate equation.