Question

A thin circular disk is in the xy plane as shown in the figure. The ratio of its moment of inertia about z and z' axes will be

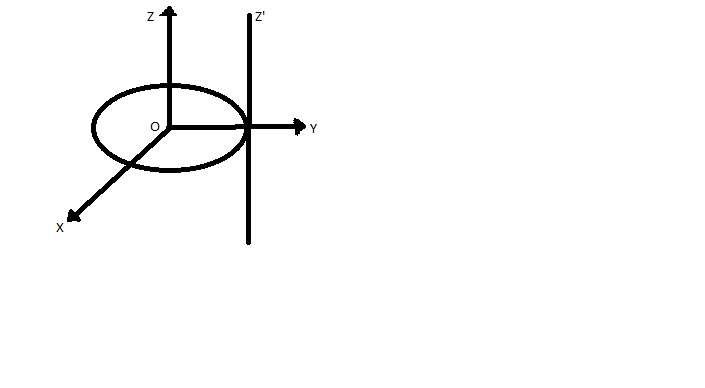
A 1:2
B 1:4
C 1:3
D 1:5

Answer 1

Hint: The moment of inertia of the circular disc about an axis passing through the centre (IC) and perpendicular to the plane of the disc is\[\dfrac{{M{R^2}}}{2}\]. Now by using the parallel axis theorem we could find the moment of inertia of the disc about the axis z'. The moment of inertia about the axis z' is I=IC+Mh$^2$where, IC= Moment of inertia about the centre.
M = mass of the body
h =perpendicular distance between both the axis.

Complete step by step answer:
We know that the moment of inertia of the circular disc about an axis passing through the centre and perpendicular to the plane of the disc is\[\dfrac{{M{R^2}}}{2}\].
Now to find the MI of the disc about the axis said we use the parallel axis theorem.
Therefore MI about the axis z’ can be written as I=IC+Mh$^2$
$ \Rightarrow $I=\[\dfrac{{M{R^2}}}{2}\]+MR$^2$=$\dfrac{{3M{R^2}}}{2}$
Taking the ratio of IC and I,
$\dfrac{{{I_C}}}{I} = \dfrac{{\dfrac{{M{R^2}}}{2}}}{{\dfrac{{3M{R^2}}}{2}}} = \dfrac{1}{3}$
Therefore the required ratio is $\dfrac{1}{3}$.

So, the correct answer is “Option A”.

Additional Information:
A quantity expressing a body's tendency to resist angular acceleration, which is the sum of the products of the mass of each particle in the body with the square of its distance from the axis of rotation is called moment of inertia.
Parallel axis theorem: The moment of inertia of a body about an axis parallel to the body passing through its centre is equal to the sum of moment of inertia of body about the axis passing through the centre and product of mass of the body times the square of distance between the two axes.

Note:
Students should know how to derive the MI of simple geometrical figures to solve such types of problems. To calculate the moment of inertia about any axis we can use the parallel axis theorem and perpendicular axis theorem.