Question

A transverse wave is represented by the equation, $y={{y}_{o}}\sin \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right)$. For what value of $\lambda $ is the maximum particle velocity equal to two times the wave velocity?
$\begin{align}
  & \text{A}\text{. }\lambda =\pi {{y}_{o}} \\
 & \text{B}\text{. }\lambda =\dfrac{\pi {{y}_{o}}}{2} \\
 & \text{C}\text{. }\lambda =\dfrac{\pi {{y}_{o}}}{3} \\
 & \text{D}\text{. }\lambda =\dfrac{2\pi }{{{y}_{o}}} \\
\end{align}$

Answer 1

Hint: Transverse wave is a type of motion in which all points on a wave oscillate along the paths that are at the right angles to the direction of the wave's advance. Wave velocity is the distance travelled by wave in unit time while particle velocity is the distance travelled by a particle of moving wave in unit time. We will find the wave velocity and particle velocity of the given wave and determine the value of $\lambda $ when the particle velocity equals two times the wave velocity.

Complete step by step answer:
A transverse wave is a moving wave in which the oscillations of particles are perpendicular to the direction of the propagation of the wave.

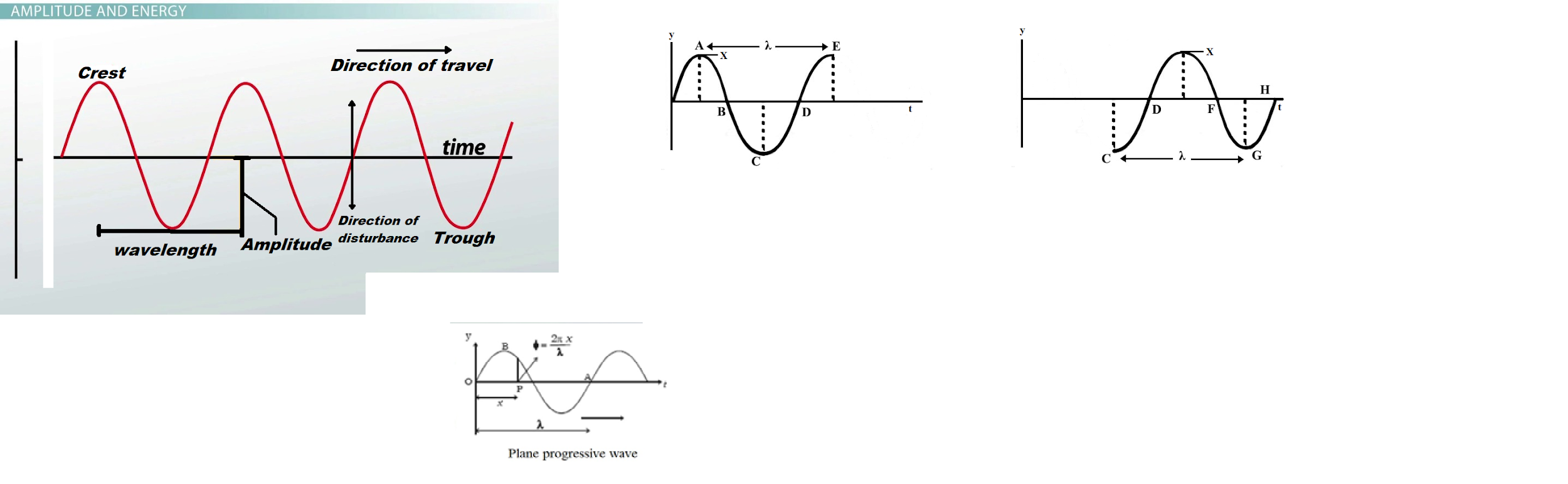
Expression for a transverse wave;
$y={{y}_{o}}\sin \left[ 2\pi \left( ft-\dfrac{x}{\lambda } \right) \right]$
Where,
$y$ is the current displacement of particle of wave
${{y}_{o}}$ is the maximum displacement of particle of wave
$f$ is the frequency of wave
$t$ is the time
$x$ is the distance of particle from the origination of wave
$\lambda $ is the wavelength
Speed of a transverse wave or Wave speed is defined as the distance a wave travels per unit time.
Particle velocity is defined as the velocity of a particle, be it real or imagined, in a medium as it transmits a wave.
We are given a transverse wave having equation $y={{y}_{o}}\sin \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right)$ and we need to find the value of $\lambda $ when the particle velocity equal to two times the wave velocity.
Wave velocity is given by $v$
Particle velocity is given by,
$\dfrac{dy}{dt}=\dfrac{d}{dt}\left[ {{y}_{o}}\sin \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right) \right]$
We get,
\[\begin{align}
  & \dfrac{dy}{dt}={{y}_{o}}\cos \left[ \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right) \right]\times \dfrac{d}{dt}\left[ \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right) \right] \\
 & \dfrac{dy}{dt}={{y}_{o}}\cos \left[ \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right) \right]\times \left( \dfrac{2\pi }{\lambda } \right)\times v \\
\end{align}\]
For maximum particle velocity,
\[\cos \left[ \left( \dfrac{2\pi }{\lambda } \right)\left( vt-x \right) \right]=1\]
Therefore,
${{\left( \dfrac{dy}{dt} \right)}_{\max }}={{y}_{o}}\dfrac{2\pi v}{\lambda }$
We have,
Maximum particle velocity is double of the wave velocity,
${{v}_{P\left( \max \right)}}=2v$
Putting values,
${{v}_{P\left( \max \right)}}={{y}_{o}}\dfrac{2\pi v}{\lambda }$
We get,
$\begin{align}
  & {{y}_{o}}\dfrac{2\pi v}{\lambda }=2v \\
 & \lambda ={{y}_{o}}\pi \\
\end{align}$
The value of $\lambda $ when the maximum velocity equals double the wave velocity is $\pi {{y}_{o}}$
Hence, the correct option is A.

Note:
Students should not get confused between particle velocity and wave velocity. In the above question, we were given the relation between particle velocity and wave velocity. For calculating particle velocity we can also use the formula, ${{v}_{P}}=-v\dfrac{dy}{dx}$, where, ${{v}_{P}}$ is the particle velocity and $v$ is the wave velocity. Also, it should be kept in mind that the velocity of the wave is the division of wavelength and time period, thus, velocity of a progressive wave can be expressed as the coefficient of $t$ divided by coefficient of $x$ as given in the wave equation.