
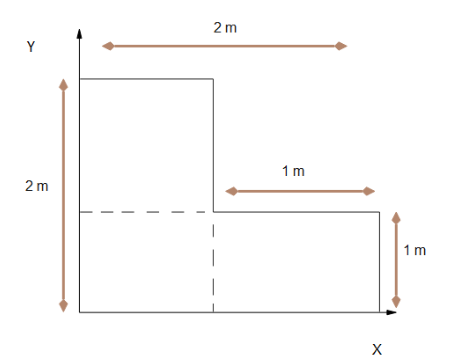
A uniform L shaped lamina with dimensions is shown. The mass of the lamina is

Answer
416.4k+ views
Hint:To solve this we need to know the basic formulas of center of mass. In this problem we can see there are three discrete bodies each are rectangles. We have to find the center of mass of each and combine using the standard formula of center of mass , and we will get the answer.
Formula used:
Centre of Mass formula:
Complete step by step answer:
To make things simple we would consider the given body on a coordinate system. Now we can get the coordinates of the vertices of the given body. As the three laminas are uniform, by the help of the coordinates, we can see that the three laminas are squares , each of mass 1 kg. Let the center of mass of the three bodies Be C_1, C_2, C_3 respectively. As all three are square, their COM will be their mid points, thus
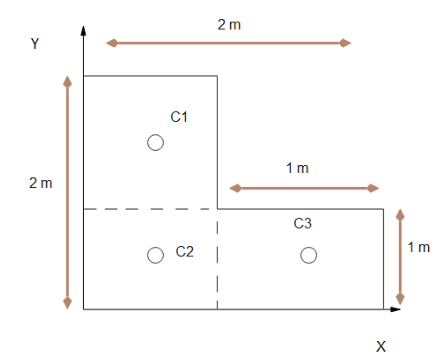
Now we have found the Center of mass of discrete bodies, now let’s apply the formula of Center of mass for a combined body :-
For X axis Center of mass:
Now for Centre of mass along Y axis:
Thus the centre of mass of the L shaped lamina of
Note:These questions require only the centre of mass formula to solve them and generally are very easy. It is important to remember the centre of mass formula for each body (students often get confused between the formulas of centre of mass). The more complex problems would require integrating the mass of the body, which we can do by considering a small strip from the body. We can also solve the given problem by method of integration.
Formula used:
Centre of Mass formula:
Complete step by step answer:
To make things simple we would consider the given body on a coordinate system. Now we can get the coordinates of the vertices of the given body. As the three laminas are uniform, by the help of the coordinates, we can see that the three laminas are squares , each of mass 1 kg. Let the center of mass of the three bodies Be C_1, C_2, C_3 respectively. As all three are square, their COM will be their mid points, thus

Now we have found the Center of mass of discrete bodies, now let’s apply the formula of Center of mass for a combined body :-
For X axis Center of mass:
Now for Centre of mass along Y axis:
Thus the centre of mass of the L shaped lamina of
Note:These questions require only the centre of mass formula to solve them and generally are very easy. It is important to remember the centre of mass formula for each body (students often get confused between the formulas of centre of mass). The more complex problems would require integrating the mass of the body, which we can do by considering a small strip from the body. We can also solve the given problem by method of integration.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




