
A uniform rod of weight
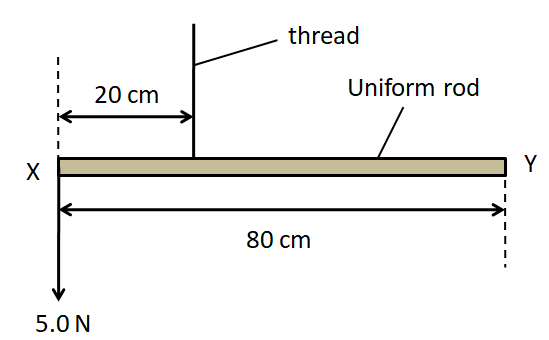
(A)
(B)
(C)
(D)

Answer
482.1k+ views
Hint To solve this question, we need to consider the vertical equilibrium of the given rod to determine the tension in the thread. Then, the consideration of the rotational equilibrium of the same rod will give out the required answer.
Complete step by step answer
Let the tension in the thread be
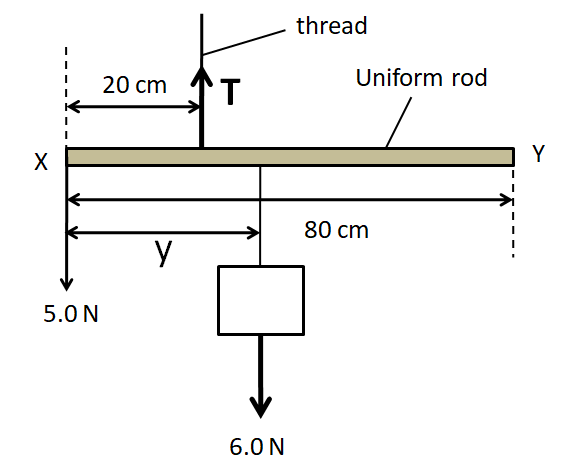
Now we consider the free body diagram of the rod.
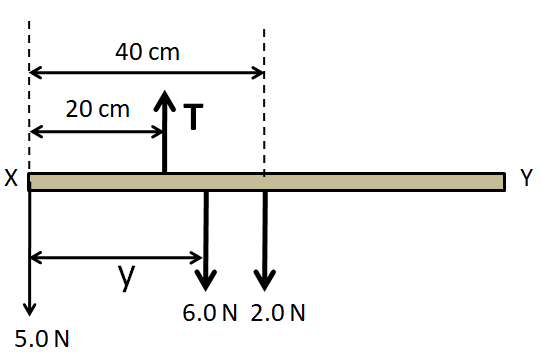
We know that the weight of a body acts on its centre of gravity. As the centre of gravity of a rectangular uniform rod is located at its midpoint so the weight
Now, we consider the rotational equilibrium of the rod at the end X of the rod. Summing up the moments of all the forces about the point X, we get
From (1)
Dividing both sides by
Thus, the distance of the
Hence the correct answer is option D.
Note
We could have got the correct answer to this question even without determining the value of the tension in the thread also. We know that if a body is in rotational equilibrium, then the net torque about any point on it is equal to zero. Therefore, we can balance the torque at the point, where the rod is suspended by the thread so that the torque due to the force of tension in the thread would become zero. In this way we can solve these types of problems more quickly.
Complete step by step answer
Let the tension in the thread be

Now we consider the free body diagram of the rod.

We know that the weight of a body acts on its centre of gravity. As the centre of gravity of a rectangular uniform rod is located at its midpoint so the weight
Now, we consider the rotational equilibrium of the rod at the end X of the rod. Summing up the moments of all the forces about the point X, we get
From (1)
Dividing both sides by
Thus, the distance of the
Hence the correct answer is option D.
Note
We could have got the correct answer to this question even without determining the value of the tension in the thread also. We know that if a body is in rotational equilibrium, then the net torque about any point on it is equal to zero. Therefore, we can balance the torque at the point, where the rod is suspended by the thread so that the torque due to the force of tension in the thread would become zero. In this way we can solve these types of problems more quickly.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

The reason why India adopted the policy of nonalignment class 11 social science CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

The plastids which are coloured green and colourless class 11 biology CBSE




