
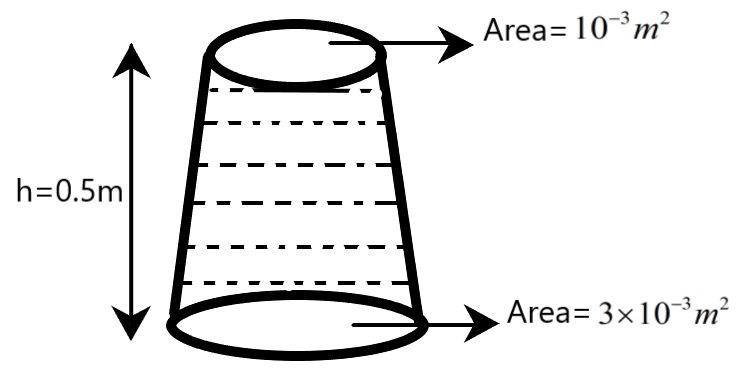
A uniformly tapering vessel shown in figure is filled with a liquid of density

Answer
493.2k+ views
1 likes
Hint: Pressure is defined as force per unit area. Pressure on the base of a vessel containing liquid is also defined as the product of density of liquid, height of the liquid in the vessel and the acceleration due to gravity. Combining both these definitions, we can arrive at the pressure on the base of a vessel, containing liquid.
Formula used:
Complete answer:
We are provided with a uniformly tapering vessel, filled with a liquid of density
We know that pressure is defined as force per unit area. Mathematically, pressure is given by
where
Let this be equation 1.
When we consider a vessel containing liquid, we also know that pressure on the base of the vessel is equal to the product of density of liquid, height of the liquid in the vessel and the acceleration due to gravity. If
where
Let this be equation 2.
Combining equation 1 and equation 2, we have
Let this be equation 3.
Using equation 3, we can determine the force acting on the base of the given tapering vessel containing liquid as follows:
where
So, the correct answer is “Option A”.
Note:
Students need to notice that the area on top of the tapering vessel, as given in the figure, is irrelevant in the above problem. We are asked to determine the force acting on the base of the vessel, and not the force acting on the top of the vessel. Therefore, area of base of the vessel, density of the liquid and height of liquid in the vessel are the only important parameters to be used from the given diagram.
Formula used:
Complete answer:
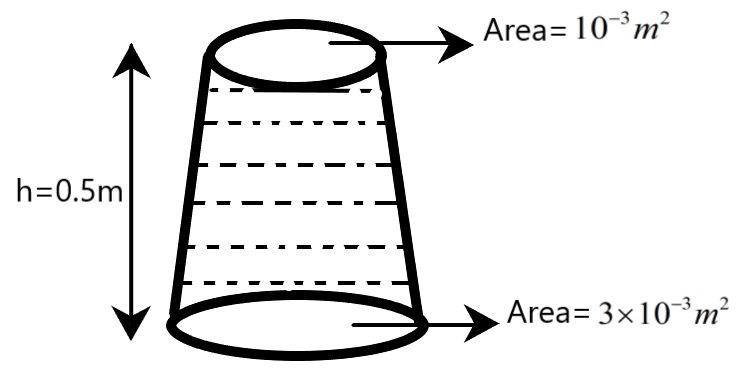
We are provided with a uniformly tapering vessel, filled with a liquid of density

We know that pressure is defined as force per unit area. Mathematically, pressure is given by
where
Let this be equation 1.
When we consider a vessel containing liquid, we also know that pressure on the base of the vessel is equal to the product of density of liquid, height of the liquid in the vessel and the acceleration due to gravity. If
where
Let this be equation 2.
Combining equation 1 and equation 2, we have
Let this be equation 3.
Using equation 3, we can determine the force acting on the base of the given tapering vessel containing liquid as follows:
where
So, the correct answer is “Option A”.
Note:
Students need to notice that the area on top of the tapering vessel, as given in the figure, is irrelevant in the above problem. We are asked to determine the force acting on the base of the vessel, and not the force acting on the top of the vessel. Therefore, area of base of the vessel, density of the liquid and height of liquid in the vessel are the only important parameters to be used from the given diagram.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




