
What is a vertical asymptote in calculus?
Answer
441k+ views
Hint: To solve this question you should know about the asymptotes or vertical asymptotes. Any vertical asymptote is a place where the function is undefined and the limit of that function does not exist. This is happening because as 1 approaches to the asymptote, even any small shift in the x – value leads to arbitrarily large fluctuations in the value of the functions.
Complete step by step answer:
According to our question we have to explain about the vertical asymptote in calculus.
So, in calculus a vertical asymptote is a place where the function is undefined and the limits of that function does not exist.
In simple words we can say that –
“Definition : The line
In this definition the superscript + denotes the right hand limits of
If we take an example and consider the function
In this condition two of our statements are true from the definition. Especially the fourth and first. Therefore, we can say that:
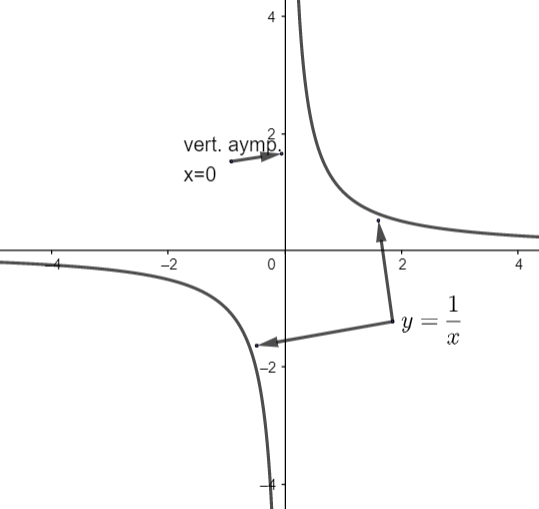
Note: For any vertical asymptote point it is compulsory that it is satisfying at least any one point which is defined in the definition. If that is not satisfying any point then it can’t be possible to be a vertical asymptote at that point in that function’s curve. And this is mandatory for every function.
Complete step by step answer:
According to our question we have to explain about the vertical asymptote in calculus.
So, in calculus a vertical asymptote is a place where the function is undefined and the limits of that function does not exist.
In simple words we can say that –
“Definition : The line
In this definition the superscript + denotes the right hand limits of
If we take an example and consider the function
In this condition two of our statements are true from the definition. Especially the fourth and first. Therefore, we can say that:

Note: For any vertical asymptote point it is compulsory that it is satisfying at least any one point which is defined in the definition. If that is not satisfying any point then it can’t be possible to be a vertical asymptote at that point in that function’s curve. And this is mandatory for every function.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




