
A wet open umbrella is held vertical and whirled about the handle at a uniform rate of 21 revolutions in 44s. If the rim of the umbrella is a circle of 1m in diameter and the height of the rim above the floor is 4.9m, the locus of the drop is a circle of radius.
Answer
504.6k+ views
Hint:
The problem is based on circular motion. The concepts of angular velocity
Step by step solution:
Let’s start by making a diagram of the top view of the umbrella.
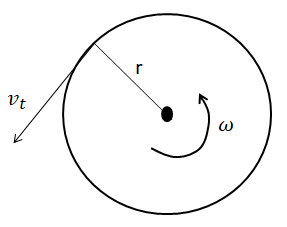
Given in the problem, we have the umbrella being whirled at 21 revolutions in 44s. Hence, the angular velocity of the umbrella is,
The tangential velocity of the umbrella being whirled is given by,
Hence, the tangential velocity of the umbrella is,
Now, considering the drop, that falls from the edge of the rim to the floor, since the drop was at the edge of the rim, the drop was given horizontal velocity (which is the tangential velocity) only. Below is a diagram of the vertical view of the umbrella.
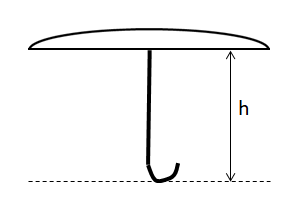
Hence, the drop was not having vertical velocity initially.
That is,
Hence, the time of flight for the drop is, 1s.
Now, let’s consider the drop travelling a distance x, before hitting the ground, from the time it left the umbrella. That flight time is equal to 1s, as found earlier. The locus of all such points at a distance x, from the perimeter of the umbrella will be another circle of radius R, taking the same center as that of the umbrella. Below is a diagram to understand better.
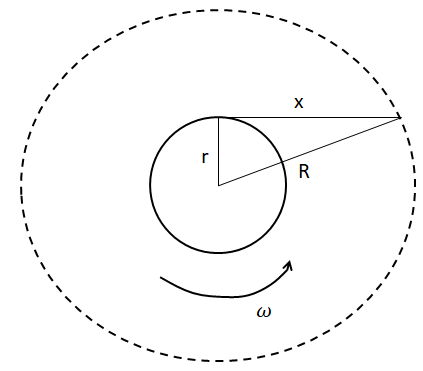
Here, a right angled triangle is formed, with R as the hypotenuse. (x) is the distance travelled by the drop before hitting the ground. The drop has horizontal velocity of
There isn’t any linear horizontal acceleration acting on the drop, hence, a=0. Hence using the Newton’s law of motion,
Using the Pythagorean theorem for the right angled triangle,
Hence, the locus of all the drops will form a circle of radius
Note:
It’s necessary to make the diagram, so that you don’t make mistakes in making the right angled triangle towards the ending and don’t end up having wrong values for the wrong sides of the right angled triangle. The ending part, where the 2 consecutive circles are there with the common center is the part, where one should be more careful.
The problem is based on circular motion. The concepts of angular velocity
Step by step solution:
Let’s start by making a diagram of the top view of the umbrella.

Given in the problem, we have the umbrella being whirled at 21 revolutions in 44s. Hence, the angular velocity of the umbrella is,
The tangential velocity of the umbrella being whirled is given by,
Hence, the tangential velocity of the umbrella is,
Now, considering the drop, that falls from the edge of the rim to the floor, since the drop was at the edge of the rim, the drop was given horizontal velocity (which is the tangential velocity) only. Below is a diagram of the vertical view of the umbrella.

Hence, the drop was not having vertical velocity initially.
That is,
Hence, the time of flight for the drop is, 1s.
Now, let’s consider the drop travelling a distance x, before hitting the ground, from the time it left the umbrella. That flight time is equal to 1s, as found earlier. The locus of all such points at a distance x, from the perimeter of the umbrella will be another circle of radius R, taking the same center as that of the umbrella. Below is a diagram to understand better.

Here, a right angled triangle is formed, with R as the hypotenuse. (x) is the distance travelled by the drop before hitting the ground. The drop has horizontal velocity of
There isn’t any linear horizontal acceleration acting on the drop, hence, a=0. Hence using the Newton’s law of motion,
Using the Pythagorean theorem for the right angled triangle,
Hence, the locus of all the drops will form a circle of radius
Note:
It’s necessary to make the diagram, so that you don’t make mistakes in making the right angled triangle towards the ending and don’t end up having wrong values for the wrong sides of the right angled triangle. The ending part, where the 2 consecutive circles are there with the common center is the part, where one should be more careful.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




