
(A)
(B)
(C)
(D)
Answer
476.1k+ views
Hint: As we know that the body centred cubic lattice is a unit cell in which number of atoms are present at eight corners as well as at the centre of the body. Distance between oppositely charged ions in the lattice can be calculated by calculating the radius of the atom.
Complete step by step answer:
As we know that the relation of edge length and radius of atom is calculated by face diagonal and body diagonal as shown below.
Where
In this way, by putting the value of edge length we can calculate radius of atom as
Now we know that the distance is the closest approach of two atoms so the relation between radius of atom and distance is as
Now we put the value of
Note: The total number of atoms in a body centred cubic unit cell is 2 in which one from body centre and one from eight corners. The central atom in the body centred cubic lattice makes a body diagonal which can be calculated by face diagonal and the edge of the body centred cubic lattice by Pythagoras theorem. The body central atom also measures the distance of closest approach as we can see in the below diagram.
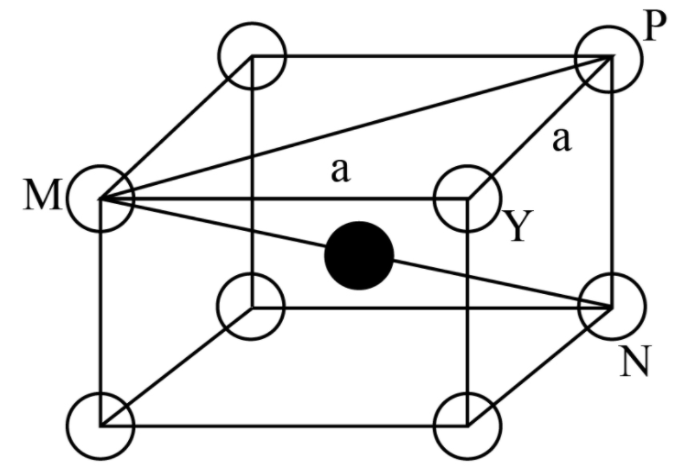
As the spheres at the body centre touches the spheres at the corners, body diagonal,
Further, face diagonal,
And body diagonal,
Therefore,
Or
Complete step by step answer:
As we know that the relation of edge length and radius of atom is calculated by face diagonal and body diagonal as shown below.
Where
In this way, by putting the value of edge length we can calculate radius of atom as
Now we know that the distance is the closest approach of two atoms so the relation between radius of atom and distance is as
Now we put the value of
Note: The total number of atoms in a body centred cubic unit cell is 2 in which one from body centre and one from eight corners. The central atom in the body centred cubic lattice makes a body diagonal which can be calculated by face diagonal and the edge of the body centred cubic lattice by Pythagoras theorem. The body central atom also measures the distance of closest approach as we can see in the below diagram.

As the spheres at the body centre touches the spheres at the corners, body diagonal,
Further, face diagonal,
And body diagonal,
Therefore,
Or
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




