
ABC is a right angled triangle right angled at B such that BC = 6 cm and AB = 8 cm. A circle with center O is inscribed in triangle ABC. The radius of the circle is
A. 1cm
B. 2cm
C. 3cm
D. 4cm
Answer
521.1k+ views
1 likes
Hint: In these types of questions remember the Pythagoras theorem i.e.
Complete step-by-step answer:
Let’s use the given information and draw a rough diagram
Let’s draw a right angle triangle with a circle inside it with center O.
Then draw a line OE and OF which is perpendicular to BC and AB and a line OB
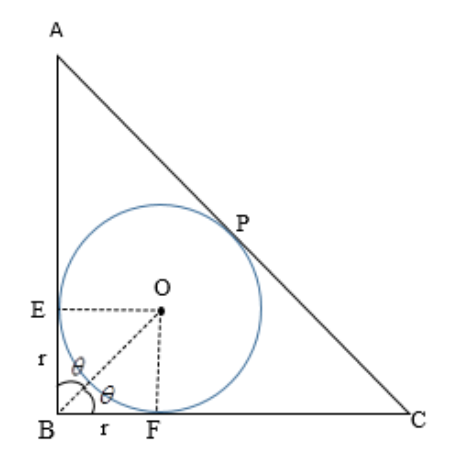
First let’s find the value of AC
By the Pythagoras theorem
Applying both side by square root
By the theorem of Tangent to a circle i.e. if two tangents are drawn from an external point of the circle, then they are of equal lengths therefore BE = BF, CP = CF and AP = AE.
By the above statement BE = BF = r
And CP = CF
CP = CF
Now putting the value of BF and BE in the equation 1
AC – AE = BC – BF since AP=AE by the theorem of tangent to a circle
AC – AE = BC – BF (equation 2)
Putting the value of AC, AE, BC and BF in equation 2
10 – (6 – r) = 8 – r
Therefore the radius of the circle inside the triangle is 2cm.
Note: In these types of questions first draw a rough diagram then find the unknown value of side of the triangle then construct lines from the center of the circle OF and OE that are perpendicular to the line AB and BC also a line OB which bisect the BE and BF then with the help theorem of tangent to a circle show that AP = AE, CP = CF and BE = BF then use the statement CP = CF and put the values of CP and CF and find the value of radius of circle.
Complete step-by-step answer:
Let’s use the given information and draw a rough diagram
Let’s draw a right angle triangle with a circle inside it with center O.
Then draw a line OE and OF which is perpendicular to BC and AB and a line OB

First let’s find the value of AC
By the Pythagoras theorem
Applying both side by square root
By the theorem of Tangent to a circle i.e. if two tangents are drawn from an external point of the circle, then they are of equal lengths therefore BE = BF, CP = CF and AP = AE.
By the above statement BE = BF = r
And CP = CF
CP = CF
Now putting the value of BF and BE in the equation 1
AC – AE = BC – BF since AP=AE by the theorem of tangent to a circle
AC – AE = BC – BF (equation 2)
Putting the value of AC, AE, BC and BF in equation 2
10 – (6 – r) = 8 – r
Therefore the radius of the circle inside the triangle is 2cm.
Note: In these types of questions first draw a rough diagram then find the unknown value of side of the triangle then construct lines from the center of the circle OF and OE that are perpendicular to the line AB and BC also a line OB which bisect the BE and BF then with the help theorem of tangent to a circle show that AP = AE, CP = CF and BE = BF then use the statement CP = CF and put the values of CP and CF and find the value of radius of circle.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




