
Answer
535.8k+ views
Hint: Draw perpendicular from one of the vertices to the side opposite to the vertex. Use the fact that the angles opposite to equal sides are equal to find the value of each angle of the equilateral triangle. Use trigonometric properties to relate the length of any one of the sides to the length of the altitude.
Complete step-by-step answer:
We have an equilateral triangle
Consider the equilateral triangle
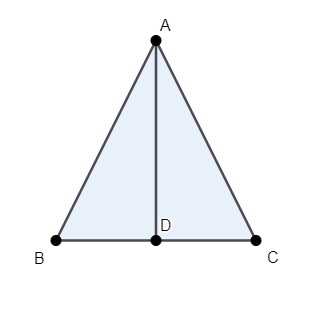
We know that the angles opposite to equal sides are equal.
As all the sides of an equilateral triangle are equal, the angles opposite to them are equal.
Thus, all the angles of an equilateral triangle are equal. Let’s assume this angle to be
As the sum of all the angles of a triangle is
Thus, the measure of each of the angles of an equilateral triangle is
To find the length of altitude
We will consider the triangle
Using the property of sine function in a right angled triangle
We know that
Rearranging the terms, we have
Hence, the length of each of the altitudes is
Note: One must clearly know the meaning of terms equilateral triangle and altitude along with trigonometric functions. Equilateral triangle is a triangle in which the measure of all the sides is equal. Altitude of a triangle is a line segment through a vertex perpendicular to the side opposite to the vertex.
Complete step-by-step answer:
We have an equilateral triangle
Consider the equilateral triangle

We know that the angles opposite to equal sides are equal.
As all the sides of an equilateral triangle are equal, the angles opposite to them are equal.
Thus, all the angles of an equilateral triangle are equal. Let’s assume this angle to be
As the sum of all the angles of a triangle is
Thus, the measure of each of the angles of an equilateral triangle is
To find the length of altitude
We will consider the triangle
Using the property of sine function in a right angled triangle
We know that
Rearranging the terms, we have
Hence, the length of each of the altitudes is
Note: One must clearly know the meaning of terms equilateral triangle and altitude along with trigonometric functions. Equilateral triangle is a triangle in which the measure of all the sides is equal. Altitude of a triangle is a line segment through a vertex perpendicular to the side opposite to the vertex.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




