
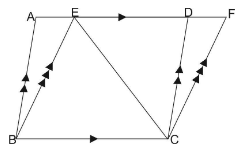
ABCD and BCEF are parallelograms. If area of

Answer
481.8k+ views
Hint: In this problem we first find area of parallelogram BCFE from given area of triangle BCE and then using area of triangle we find height of triangle and then using this height we will find area of parallelogram ABCDE and hence the required solution of the problem .
Formulas used: Area of parallelogram =
Complete step by step solution:
It is given an area of triangle EBC =
Also, triangle EBC is drawn on common base BC of parallelogram BCFE and diagonal EC divides parallelogram BCFE in two equal parts or areas.
Therefore, area of ||gm BCFE =
Hence, from above we see that area of ||gm(BCFE) is
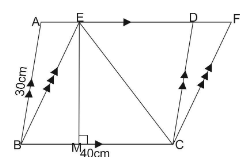
Also, we know that area of triangle BCE is given as:
Substituting values in above we have,
Therefore, height of triangle BCE or ||gm BCDA is
Therefore, area of ||gm BCDA is given as:
Substituting values in above. We have
Hence, from above we can say that area of parallelogram ABCD is
So, the correct answer is “Option A”.
Note: We can also find the solution of a given problem by using the concept of theorem. In this we know that the area of two parallelograms are equal if they lie on the same base between parallel lines and the area of the triangle is half of the area of the parallelogram if the triangle and parallelogram have the same base and in between parallel lines. So, form this concept from the area of a given triangle we can easily find the area of one parallelogram and then form one parallelogram we can find the area of the other parallelogram and hence the required solution of the problem.
Formulas used: Area of parallelogram =
Complete step by step solution:
It is given an area of triangle EBC =
Also, triangle EBC is drawn on common base BC of parallelogram BCFE and diagonal EC divides parallelogram BCFE in two equal parts or areas.
Therefore, area of ||gm BCFE =
Hence, from above we see that area of ||gm(BCFE) is

Also, we know that area of triangle BCE is given as:
Substituting values in above we have,
Therefore, height of triangle BCE or ||gm BCDA is
Therefore, area of ||gm BCDA is given as:
Substituting values in above. We have
Hence, from above we can say that area of parallelogram ABCD is
So, the correct answer is “Option A”.
Note: We can also find the solution of a given problem by using the concept of theorem. In this we know that the area of two parallelograms are equal if they lie on the same base between parallel lines and the area of the triangle is half of the area of the parallelogram if the triangle and parallelogram have the same base and in between parallel lines. So, form this concept from the area of a given triangle we can easily find the area of one parallelogram and then form one parallelogram we can find the area of the other parallelogram and hence the required solution of the problem.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE





