Question

ABCD is a circle and circles are drawn with AO, CO, DO, and OB as diameters. Areas “X” and “Y” are labeled, what is the value of $\dfrac{X}{Y}$?

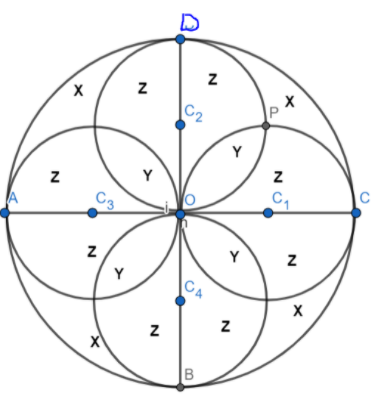
A). 1
B). $\dfrac{1}{2}$
C). $\dfrac{1}{4}$
D). $\dfrac{\pi }{4}$

Answer 1

Hint: As you see from the figure all areas had been marked. The first thing that you have to consider the areal symmetry of the circle. But the only problem is the portion in the $\overset\frown{OCD}$ part as if you subtract the area of a small circle [whose diameter is OC and OD], then you basically subtract the \[\overset\frown{OP}\] twice.

Complete step-by-step solution:

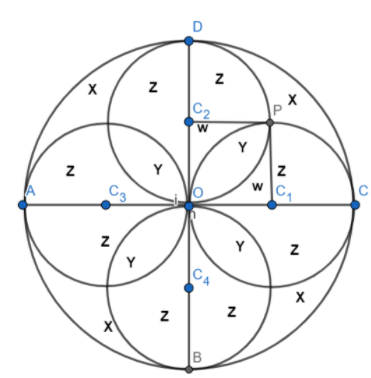
Here we introduce a new areal portion “w”
\[\overleftrightarrow{O{{C}_{1}}}=\overleftrightarrow{O{{C}_{2}}}=a\] and \[\square O{{C}_{1}}P{{C}_{2}}\] is square.
First , see the point P , and join \[\overleftrightarrow{{{C}_{_{2}}}P}\] and \[\overleftrightarrow{{{C}_{1}}P}\]
After joining “\[\square O{{C}_{1}}P{{C}_{2}}\]” become a square.
Now suppose that the radius of the big circle is “2a” unit.
so the radius of the small circle is “a” unit. {as mentioned in the question}.
As you may know, a circle whose radius is “r “ unit has an area
\[A=\pi {{r}^{2}}\] sq. unit .
In this way , we can calculate the area of big and small circle ,
Area of big circle : \[\pi {{(2a)}^{2}}=4\pi {{a}^{2}}\]
 Similarly area of small circle is : \[\pi {{a}^{2}}\]
As see from the figure we have to consider only \[\dfrac{1}{4}\]th area of big circle i.e.
\[area\text{ }(\overset\frown{OCD})\] : \[\dfrac{1}{4}(4\pi {{a}^{2}})=\pi {{a}^{2}}\]
Now we calculate the area of the square \[\square O{{C}_{1}}{{C}_{2}}P\], as \[\overleftrightarrow{O{{C}_{1}}}=\overleftrightarrow{O{{C}_{2}}}=a\],
so the length of each side of the square is “a” unit . So the area will be \[{{a}^{2}}\] sq.unit .
Now join \[\overleftrightarrow{{{C}_{1}}P}\], clearly after joining the circle is partitioned into two equal half-circle of equal area.
The area of each half circle will be \[\dfrac{1}{2}.\dfrac{\pi {{a}^{2}}}{2}=\dfrac{\pi {{a}^{2}}}{4}\] sq.unit .
Here we “w” is the area of the portion of half-circle and “y” is the area of closed-loop of OP.
 Next , we will try to formulate the linear equation ,
\[\begin{align}
  & 2w+y={{a}^{2}}\text{....................................(1)} \\
 & w+y=\dfrac{\pi {{a}^{2}}}{4}\text{.............................(2)} \\
\end{align}\]
after substituting the value from (2) and put it on (1) ,we get \[y={{a}^{2}}\left( \dfrac{\pi }{2}-1 \right)\]
To get the value x we need to solve following equation ,
\[x+{{a}^{2}}+\dfrac{\pi {{a}^{2}}}{2}=\pi {{(2a)}^{2}}\dfrac{1}{4}\]
\[\Rightarrow x={{a}^{2}}\left( \dfrac{\pi }{2}-1 \right)\]
This implies \[\dfrac{X}{Y}=1\]
Hence option (a) is correct.

Note: Please do not try to use the method of definite integral, by assuming the equation of the circle. In order to apply, we have to assume the coordinate and equation of the circle, solving, and manipulating this type of equation is a very tedious process.