Question

ABCD is a rectangle in which diagonal AC bisects $\angle A$ as well $\angle C$.
Show that,
ABCD is square.
Diagonal BD bisects $\angle B$ as well as $\angle D$.

Answer 1

Hint: As we know diagonal bisects $\angle A$ and $\angle C$, so use the fact that measurement of each angle is ${{90}^{\circ }}$ and then use the fact that equal sides have equal opposite angles of triangle.

Complete step-by-step answer:
In the question it is given that ABCD is a rectangle which diagonal bisects $\angle A,\angle C$.
So now in a rectangle ABCD, AC is joined.
 

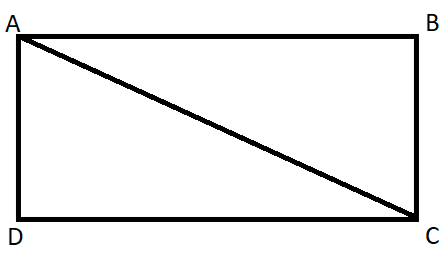
In the question it is already given that AC bisects $\angle A$ which means
$\angle DAC=\angle CAB=x$
So we can say that
$x+x={{90}^{\circ }}$ as $\angle A={{90}^{\circ }}$ which is a property of the rectangle.
So $x={{45}^{\circ }}$ which means that
$\angle DCA=\angle CAB={{45}^{\circ }}$
Similarly, AC bisects $\angle C$ which means
$\angle DCA=\angle BCA$
Let $\angle DCA=\angle BCA=y$
So we can say that
$y+y={{90}^{\circ }}$ as $\angle C={{90}^{\circ }}$
Which is the property of rectangles.
So $y={{45}^{\circ }}$ which means that
$\angle DCA=\angle BCA$
As $x=y={{45}^{\circ }}$.
So, $\angle DAC=\angle DCA$ as $x=y$
So we can say that AD=DC as equal angles have equal opposite sides.
Similarly, $\angle BAC=\angle BCA$ as $x=y$
So here also we can say that AB=BC as equal angles have equal opposite sides.
By the property of the rectangle, we can also say AB=DC. Hence we can say that AB=BC=CD=DA.
So a rectangle with all sides are equal and is considered as a square which is proved.
Square also has the property that diagonals bisect the angles of the corners. So we can say BD bisects $\angle B$ and $\angle D$ .
Hence it is proved.

Note: Another approach is, it is given that the opposite angles bisect each other, then we can say that both the triangles formed are isosceles right angle triangles. We can say that as it is isosceles then its adjacent sides are equal. Rectangles with adjacent sides are equal and are considered as a square.