Question

ABCD is a rhombus. Show that diagonal AC bisects ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.

Answer 1

Hint: In order to prove that the diagonals AC and BD bisect their respective angles, we use the properties of rhombus, such as all the sides of a rhombus are equal and the diagonals of a rhombus are perpendicular to each other. We also use a property of triangles, which says the corresponding angles of equal sides of a triangle are equal,

Complete step by step solution:
Given Data,
ABCD is a rhombus
AC and BD are the diagonals of the rhombus
With respect to the given data we construct an appropriate figure of rhombus to understand the problem better and solve it accordingly.
Let us consider a rhombus ABCD with diagonals as AC and BD intersecting at the center of the rhombus O.
It looks as follows:

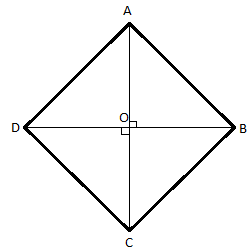
According to the property of a rhombus, all the sides of the rhombus are equal, i.e.
AB = BC = CD = DA
Also, the diagonals of a rhombus are not necessarily equal but are perpendicular to each other, i.e.
AC ⊥ BD
From the figure considering the triangle ∆ABC,
AB = BC (∵sides of the rhombus)
∠CAB = ∠ACB (∵corresponding angles of equal sides of triangle are also equal)
AO = OC (∵AC is the diagonal and O is the midpoint of the diagonal)
∠ABO = ∠CBO (∵corresponding angles of equal sides of triangle are also equal)

From the figure considering the triangle ∆ADC,
AD = DC (∵sides of the rhombus)
∠DAC = ∠DCA (∵corresponding angles of equal sides of triangle are also equal)
AO = OC (∵AC is the diagonal and O is the midpoint of the diagonal)
∠CDO = ∠ADO (∵corresponding angles of equal sides of triangle are also equal)

Hence the diagonal AC bisects ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.

Note: In order to solve this type of problems the key is to know the concept and properties of a rhombus and a triangle and also their respective formulae if needed.
We basically use the concept of equal sides of a rhombus and perpendicular diagonals, along with equal sides of triangles corresponding equal angles to prove that each angle divided by the diagonals respectively is exactly divided into two halves.
Each of the angles of the rhombus is 90°, all the angles of a rhombus are equal as well.