
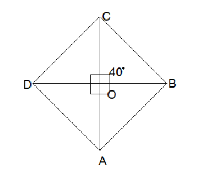
ABCD is a rhombus such that \[\angle \text{ACB}={{40}^{\circ }}\ then \\angle \text{ADB}\] is ?
A. \[{{40}^{\circ }}\]
B. \[{{50}^{\circ }}\]
C. \[{{45}^{\circ }}\]
D. None of these

Answer
453.9k+ views
Hint:To find the value of \[\angle \text{ADB}\], we first find the value of \[\angle \text{CBD}\] by using the formula of sum of all the angles equal to \[{{180}^{\circ }}\] and after finding the value of angle \[\angle \text{CBD}\], we use the alternative angle method where we find the value of \[\angle \text{ADB}\].
Complete step by step solution:
Let us draw the diagram and according to the diagram given, we will find the value of the angle \[\angle \text{CBD}\]. To find the \[\angle \text{CBD}\], we first check the triangle \[CBO\]which contains the angle \[\angle OCB\] and \[\angle COB\] i.e. \[{{40}^{\circ }}\] and \[{{90}^{\circ }}\] respectively.

Hence, the value of the angle \[\angle \text{CBD}\], we use the formula of the sum of the angle equal to \[{{180}^{\circ }}\] where we put the values in the formula as:
\[\Rightarrow \angle C+\angle O+\angle B={{180}^{\circ }}\]
\[\Rightarrow {{40}^{\circ }}+{{90}^{\circ }}+\angle B={{180}^{\circ }}\]
\[\Rightarrow \angle B={{180}^{\circ }}-{{130}^{\circ }}\]
\[\Rightarrow \angle B={{50}^{\circ }}\]
Now that we have the value of \[\angle B\], we can find the value of the \[\angle D\] by using the alternate angle method where the angle \[CBD\] and \[BDA\] are the same. Therefore, the value angle \[BDA\] is also given as \[{{50}^{\circ }}\].
Note: Rhombus like square have equal sides but the outer angles are not same hence, the outer angle of a rhombus can’t be deemed as \[{{90}^{\circ }}\] and similarly student may go wrong if they try to take the outer angles as \[{{90}^{\circ }}\] and start their questions with it.
Complete step by step solution:
Let us draw the diagram and according to the diagram given, we will find the value of the angle \[\angle \text{CBD}\]. To find the \[\angle \text{CBD}\], we first check the triangle \[CBO\]which contains the angle \[\angle OCB\] and \[\angle COB\] i.e. \[{{40}^{\circ }}\] and \[{{90}^{\circ }}\] respectively.

Hence, the value of the angle \[\angle \text{CBD}\], we use the formula of the sum of the angle equal to \[{{180}^{\circ }}\] where we put the values in the formula as:
\[\Rightarrow \angle C+\angle O+\angle B={{180}^{\circ }}\]
\[\Rightarrow {{40}^{\circ }}+{{90}^{\circ }}+\angle B={{180}^{\circ }}\]
\[\Rightarrow \angle B={{180}^{\circ }}-{{130}^{\circ }}\]
\[\Rightarrow \angle B={{50}^{\circ }}\]
Now that we have the value of \[\angle B\], we can find the value of the \[\angle D\] by using the alternate angle method where the angle \[CBD\] and \[BDA\] are the same. Therefore, the value angle \[BDA\] is also given as \[{{50}^{\circ }}\].
Note: Rhombus like square have equal sides but the outer angles are not same hence, the outer angle of a rhombus can’t be deemed as \[{{90}^{\circ }}\] and similarly student may go wrong if they try to take the outer angles as \[{{90}^{\circ }}\] and start their questions with it.
Recently Updated Pages
The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

A certain household has consumed 250 units of energy class 11 physics CBSE

The lightest metal known is A beryllium B lithium C class 11 chemistry CBSE

What is the formula mass of the iodine molecule class 11 chemistry CBSE

Trending doubts
State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

What is the modal class for the following table given class 11 maths CBSE

How do I convert ms to kmh Give an example class 11 physics CBSE

Give an example of a solid solution in which the solute class 11 chemistry CBSE




