Question

An aeroplane when $3000$ m high passes vertically above another aeroplane at an instance when their angles of elevation at the same observation point are $60^\circ $ and $45^\circ $. Find how many meters higher is this one than the other aeroplane.
A)$1350$ m B) $1268$ m C) $1000$ m D) $1160$ m

Answer 1

Hint: Here, the height of the first aeroplane is given and we have to find how high this aeroplane is from the airplane whose height is not known. First find the distance of the observation point from the aeroplanes using the angle of elevation and the height given by using the formula, $\tan \theta = \dfrac{{\text{P}}}{{\text{B}}}$. Then find the height of the other aeroplane by using the same formula. Then subtract this height from the given height and you’ll get the answer.

Complete step-by-step answer:
Given, the height of first aeroplane PS =$3000$ m whose angle of elevation from the observation point is $\angle {\text{PQS = 60}}^\circ $ .The second aeroplane has height PR= h m and the angle of elevation from observation point is $\angle {\text{PQR = 45}}^\circ $.We have to find the height of second plane from first aeroplane RS.

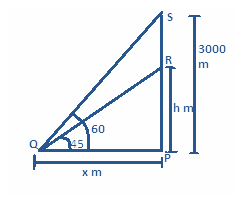
Let the distance of the observation point from both the planes PQ be x m. Then in right angled triangle SPQ,
$ \Rightarrow \tan \theta = \dfrac{{{\text{PS}}}}{{{\text{PQ}}}}$ $\left[ {{\text{as tan}}\theta {\text{ = }}\dfrac{{\text{P}}}{{\text{B}}}} \right]$
On putting the given values, we get-
$ \Rightarrow \tan {60^ \circ } = \dfrac{{3000}}{{\text{x}}} \Rightarrow \sqrt 3 = \dfrac{{3000}}{{\text{x}}}$
On rationalizing and solving for x, we get-
$ \Rightarrow {\text{x = }}\dfrac{{3000 \times \sqrt 3 }}{{\sqrt 3 \times \sqrt 3 }} = \dfrac{{3000\sqrt 3 }}{3} = 1000\sqrt 3 $ m
Now we need to find the height of the second aeroplane. So in right angled triangle RPQ,
$ \Rightarrow \tan {45^ \circ } = \dfrac{{{\text{PR}}}}{{{\text{PQ}}}}$ $ \Rightarrow 1 = \dfrac{{\text{h}}}{{\text{x}}} \Rightarrow {\text{h = x}}$
Since we know the value of x, we put it in the equation-
$ \Rightarrow {\text{h = 1000}} \times {\text{1}}{\text{.732 = 1732}}$ m
Now we have to find the height of the second aeroplane from first. So,
$ \Rightarrow {\text{RS = PS - PR}}$
On putting the given values, we get-
$ \Rightarrow {\text{RS = 3000 - 1732 = 1268}}$ m
Hence the first aeroplane is $1268$ m high from the second aeroplane.

Note: To solve this type of question, we have to draw the correct diagram. Hence it is important to read the statement carefully. In this question, the formula of $\tan \theta $ is used because one quantity is given and we have to find the other quantity and angle is also given. Here, P stands for perpendicular and B stands for base of triangle.