
An aeroplane when flying at a height of 3125 m from the ground passes vertically below another plane at an instant when the angle of elevation of the two planes from the same point on the ground are
Answer
429.6k+ views
Hint: Use trigonometric values of angles to relate the height at which the aeroplanes are flying and the distance from the point from which aeroplanes are viewed and the base from which height of aeroplane is measured. Use the fact that the base from which the height is viewed is perpendicular to the height of the aeroplane.
Complete step-by-step answer:
We have data regarding two planes flying in the same vertical line at different points. We have to find the height at which one of the planes is flying, given the other one is flying at a height of 3125 m.
Let’s assume that the two planes are viewed from point A on the ground. The plane flying at height of 3125 m is at point D and the other plane is at point C. From point A, plane at C is viewed at an angle of
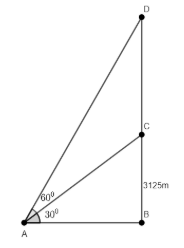
We observe that line AB is perpendicular to the vertical line BD. So, we have
In
We know that tangent of any angle is the ratio of length of perpendicular and base of the triangle.
So, we have
Thus, we have
We can rewrite the above equation as
We know that
Thus, we have
In
We know that tangent of any angle is the ratio of length of perpendicular and base of the triangle.
So, we have
Thus, we have
We can rewrite the above equation as
Substituting the value of equation (1) in above equation, we have
We know that
Thus, we have
So, we have
Hence, the distance between two planes is 6250m.
Note: One must remember the fact that the two planes are in the same vertical line and that line is perpendicular to the base from which the two aeroplanes are viewed. If we don’t consider this fact, we will get an incorrect answer.
Complete step-by-step answer:
We have data regarding two planes flying in the same vertical line at different points. We have to find the height at which one of the planes is flying, given the other one is flying at a height of 3125 m.
Let’s assume that the two planes are viewed from point A on the ground. The plane flying at height of 3125 m is at point D and the other plane is at point C. From point A, plane at C is viewed at an angle of

We observe that line AB is perpendicular to the vertical line BD. So, we have
In
We know that tangent of any angle is the ratio of length of perpendicular and base of the triangle.
So, we have
Thus, we have
We can rewrite the above equation as
We know that
Thus, we have
In
We know that tangent of any angle is the ratio of length of perpendicular and base of the triangle.
So, we have
Thus, we have
We can rewrite the above equation as
Substituting the value of equation (1) in above equation, we have
We know that
Thus, we have
So, we have
Hence, the distance between two planes is 6250m.
Note: One must remember the fact that the two planes are in the same vertical line and that line is perpendicular to the base from which the two aeroplanes are viewed. If we don’t consider this fact, we will get an incorrect answer.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




