
An arch is in the form of a parabola with its axis vertical. The arch is
Answer
411.3k+ views
Hint: A parabola is defined as a set of points that are equidistant from a directrix, which is a fixed straight line and the focus. If the parabola has directrix as the x-axis, and the focus is
Complete step by step answer:
It is given that an arch is in the form of a parabola with its axis vertical and the arch is
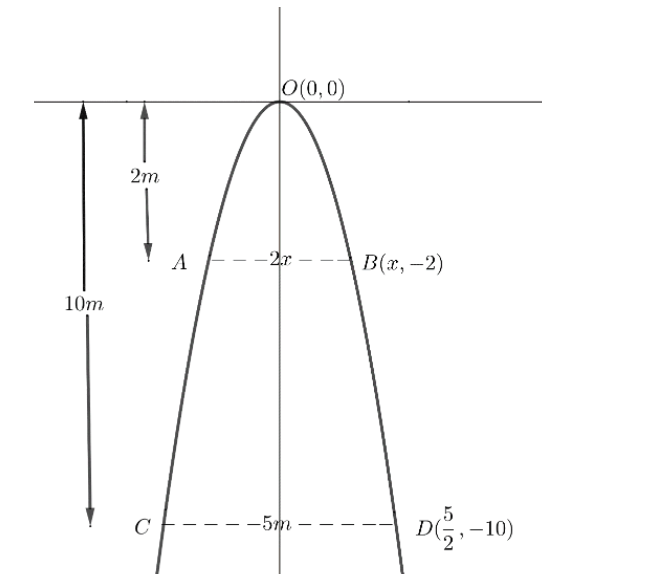
From the given figure, we see that the equation of the parabola opening on the negative y axis is given by
We need to determine the value of the focus, that is
Since the point
Substitute
So, the equation of the given parabola becomes,
Now to determine the width of the arch, when measured
Since this point
Substitute
Taking the square root on both sides of the equation
Since the width represents the length, we will not consider the negative value of
Now, since the width is
So, the width of the arc is approximately
Note:
For a parabola having the equation
Complete step by step answer:
It is given that an arch is in the form of a parabola with its axis vertical and the arch is

From the given figure, we see that the equation of the parabola opening on the negative y axis is given by
We need to determine the value of the focus, that is
Since the point
Substitute
So, the equation of the given parabola becomes,
Now to determine the width of the arch, when measured
Since this point
Substitute
Taking the square root on both sides of the equation
Since the width represents the length, we will not consider the negative value of
Now, since the width is
So, the width of the arc is approximately
Note:
For a parabola having the equation
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




