
An ellipse has OB as semi-minor axis, F and F’ are its foci and the angle FBF’ is a right angle then, the eccentricity of the ellipse is
Answer
497.7k+ views
Hint: In order to solve this problem we need to know that the Product of slopes of two perpendicular lines is -1. Drawing the diagram will help you a lot. You need to use the formula of eccentricity
Complete step-by-step answer:
The figure to this problem can be drawn as,
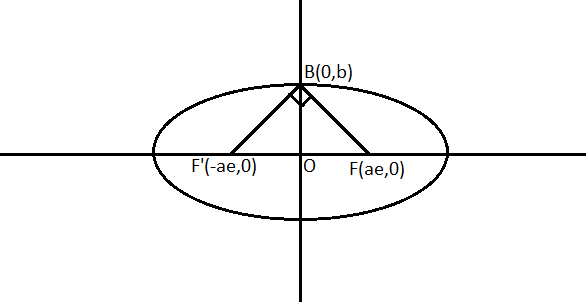
Let equation of ellipse is
We can easily see in figure coordinates of B(0, b), F (ae, 0) and F’(-ae, 0).
We know, F’B perpendicular to FB.
Thus, Product of slopes of two perpendicular lines is -1.
So, slope of F’B x slope of FB = -1
Slope of F’B =
Slope of FB =
On solving we get,
We know that
Now, put the value of
We get the new equation as,
On squaring both sides we get,
On further solving the equations we get,
So, the correct answer is “Option d”.
Note: Whenever we face such types of problems we use some important points. Like first of all draw a figure and mark coordinates then find the value of slope of lines using coordinates and as we know the product of slopes of two perpendicular lines always be -1. Knowing this will solve our problem and will give you the right answer.
Complete step-by-step answer:
The figure to this problem can be drawn as,

Let equation of ellipse is
We can easily see in figure coordinates of B(0, b), F (ae, 0) and F’(-ae, 0).
We know, F’B perpendicular to FB.
Thus, Product of slopes of two perpendicular lines is -1.
So, slope of F’B x slope of FB = -1
Slope of F’B =
Slope of FB =
On solving we get,
We know that
Now, put the value of
We get the new equation as,
On squaring both sides we get,
On further solving the equations we get,
So, the correct answer is “Option d”.
Note: Whenever we face such types of problems we use some important points. Like first of all draw a figure and mark coordinates then find the value of slope of lines using coordinates and as we know the product of slopes of two perpendicular lines always be -1. Knowing this will solve our problem and will give you the right answer.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




