
An inverted cone of height
Answer
402k+ views
Hint: We will find the volume of the cone with height of
Complete step by step answer:
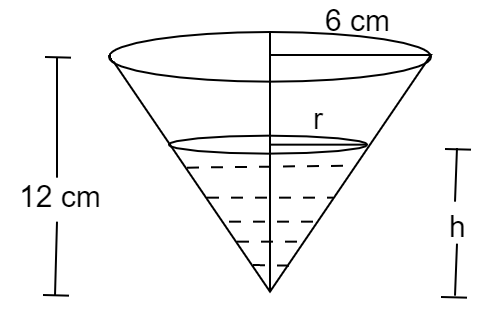
As we know,
Consider the larger cone with height
Consider the smaller cone, let its radius be
Given, the cone contains
As we know, the volume of similar figures is in the same ratio as the ratio of the cube of their heights. So, we can write
On putting the values, we get
On cross multiplication, we get
On simplification we get
Therefore, the depth of water in the cone, measured from the vertex is
Note:
We have used the concept of similar figures, but one should keep in mind that two solids are similar if and only if they are the same type of solids and their corresponding linear measures such as radii, height, base, length, etc. are proportional. Students may get confused with the height of the cone and height of the empty part of the cone.
Complete step by step answer:

As we know,
Consider the larger cone with height
Consider the smaller cone, let its radius be
Given, the cone contains
As we know, the volume of similar figures is in the same ratio as the ratio of the cube of their heights. So, we can write
On putting the values, we get
On cross multiplication, we get
On simplification we get
Therefore, the depth of water in the cone, measured from the vertex is
Note:
We have used the concept of similar figures, but one should keep in mind that two solids are similar if and only if they are the same type of solids and their corresponding linear measures such as radii, height, base, length, etc. are proportional. Students may get confused with the height of the cone and height of the empty part of the cone.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




