
An isosceles triangular plate of base 3m and altitude 3m is immersed in oil vertically with its base coinciding with the free surface of the oil of relative density 0.8. Determine the total thrust.
A. 24 KN
B. 48 KN
C. 36 KN
D. None of these
Answer
501.9k+ views
Hint: We have an isosceles triangular plate immersed in oil. To determine the total thrust, we know the equation for thrust. First, find the center of mass of the triangle and then find the thrust. Because the net thrust will be experienced at the center of mass of the plate.
Formula used:
Thrust,
Pressure,
Area,
Complete step-by-step solution:
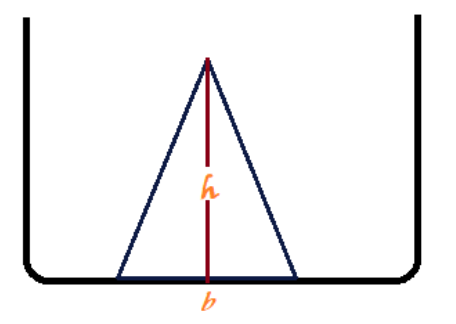
We have an isosceles triangular plate which is immersed in oil.
The base and altitude of the triangle are given in the question.
Let ‘b’ be the base of the triangle, then b = 3 m
Let ‘h’ be the altitude of the triangle, then h = 3 m.
We are also given the relative density of oil, 0.8.
Then the density of oil will be,
We need to determine the net thrust experienced by the plate.
We know that the net thrust will be experienced at the center of mass of the body.
This thrust experienced at the center of mass will be the resultant of the pressure experienced at the center of mass.
We have h= 3 m and b = 3 m.
The center of mass of the body will be at,
Therefore the center of mass of the body at 1 m distance from the free end.
Now we need to find the pressure experienced at the center of mass.
Equation of pressure is expressed as,
Here the height we consider is the height to the center of mass.
Therefore, we can write pressure as
Let us take the value of ‘g’ as
Then we have pressure at centre of mass as,
Now we need to determine the thrust.
We know the equation for thrust,
Here area is area of the isosceles triangle,
Now,
Therefore, the total thrust experienced is 36 KN.
Hence the correct answer is option C.
Note: When we keep an isosceles triangular plate in oil, the pressure will not be directly at the base of the triangular plate. The pressure will be experienced at all the points on the plate. Therefore, to find the total thrust we can find the pressure at one point on the plate and integrate it.
Formula used:
Thrust,
Pressure,
Area,
Complete step-by-step solution:

We have an isosceles triangular plate which is immersed in oil.
The base and altitude of the triangle are given in the question.
Let ‘b’ be the base of the triangle, then b = 3 m
Let ‘h’ be the altitude of the triangle, then h = 3 m.
We are also given the relative density of oil, 0.8.
Then the density of oil will be,
We need to determine the net thrust experienced by the plate.
We know that the net thrust will be experienced at the center of mass of the body.
This thrust experienced at the center of mass will be the resultant of the pressure experienced at the center of mass.
We have h= 3 m and b = 3 m.
The center of mass of the body will be at,
Therefore the center of mass of the body at 1 m distance from the free end.
Now we need to find the pressure experienced at the center of mass.
Equation of pressure is expressed as,
Here the height we consider is the height to the center of mass.
Therefore, we can write pressure as
Let us take the value of ‘g’ as
Then we have pressure at centre of mass as,
Now we need to determine the thrust.
We know the equation for thrust,
Here area is area of the isosceles triangle,
Now,
Therefore, the total thrust experienced is 36 KN.
Hence the correct answer is option C.
Note: When we keep an isosceles triangular plate in oil, the pressure will not be directly at the base of the triangular plate. The pressure will be experienced at all the points on the plate. Therefore, to find the total thrust we can find the pressure at one point on the plate and integrate it.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹42,330 per year
EMI starts from ₹3,527.5 per month
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




