
Angle in a major segment is
(A) An acute angle
(B) An obtuse angle
(C) A right triangle
(D) A reflexive angle
Answer
474.3k+ views
Hint: From the given question we have been asked to find the angle in a major segment. First we have to draw a figure which includes a minor segment
Complete step-by-step solution:
Figure:
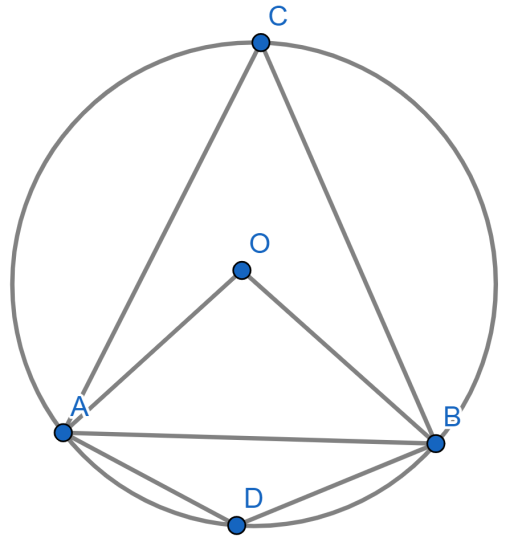
Now considering from the question we have a circle and a major and minor segment in it.
From the figure we can understand that
We know that the angle subtended by an arc of a circle at its centre is twice the angle subtended by it at any point of the alternate segment of the circle. Since
Therefore, from the figure
Therefore,
Since
Therefore,
Since the angle
Therefore,
Therefore, angle in a major segment is an acute angle.
Hence, option A is the correct option.
Note: While answering questions of this type we should surely draw a diagram and observe it carefully and apply the right concepts at the right point. And the basic concept of the circle that we used here is that the angle subtended by an arc of a circle at its centre is twice the angle subtended by it at any point of the alternate segment of the circle. Similarly by using this concept we can prove that the angle in a minor segment is an obtuse angle.
Complete step-by-step solution:
Figure:

Now considering from the question we have a circle and a major and minor segment in it.
From the figure we can understand that
We know that the angle subtended by an arc of a circle at its centre is twice the angle subtended by it at any point of the alternate segment of the circle. Since
Therefore, from the figure
Therefore,
Since
Therefore,
Since the angle
Therefore,
Therefore, angle in a major segment is an acute angle.
Hence, option A is the correct option.
Note: While answering questions of this type we should surely draw a diagram and observe it carefully and apply the right concepts at the right point. And the basic concept of the circle that we used here is that the angle subtended by an arc of a circle at its centre is twice the angle subtended by it at any point of the alternate segment of the circle. Similarly by using this concept we can prove that the angle in a minor segment is an obtuse angle.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
The highest mountain peak in India is A Kanchenjunga class 9 social science CBSE

What is the difference between Atleast and Atmost in class 9 maths CBSE

Explain the importance of pH in everyday life class 9 chemistry CBSE

On an outline map of India mark the Karakoram range class 9 social science CBSE

What is the N in statistics class 9 maths CBSE

Distinguish between Conventional and nonconventional class 9 social science CBSE




