Question

Angle inscribed in a minor segment is
(A). acute
(B). obtuse
(C). right
(D). straight

Answer 1

Hint: To solve this question, we will draw a circle and chords in it (other than diameter) and join the intersection points of the chord and the circumference of the circle to the other points of circumference.

Complete step-by-step solution -
To solve this question, we must first know what is a segment of a circle. A chord of a circle divides the circle into two regions, which are called the segments of the circle. The minor segment is the region bounded by the chord and the minor arc intercepted by chord.

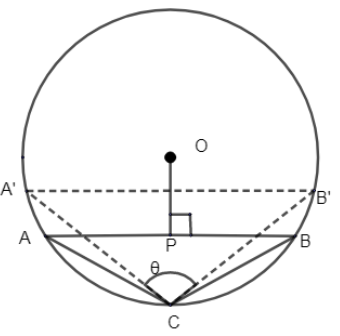
In the above figure, \[\theta \] is the angle inscribed in a minor segment. AB is any chord except the diameter of the circle. Here, we have drawn a perpendicular OP on the chord AB. We can clearly see that the \[\theta \] is less than \[{{180}^{\circ }}\] (because \[\theta \] will be \[{{180}^{\circ }}\] only in the case of straight line) and greater than \[{{90}^{\circ }}\]. Now we will move the chord AB perpendicular to OP such that the length of AB increases. Now new points are A' and B’. We will notice that the new angle formed is less than \[\theta \] but still greater than \[{{90}^{\circ }}\]. Now we will check the options one by one.
Option (a): The angle will not be acute because acute angles are less than \[{{90}^{\circ }}\] but in our case the angle is greater than \[{{90}^{\circ }}\].
Option (b): The angle will be obtuse because obtuse angles are greater than \[{{90}^{\circ }}\] and less than \[{{180}^{\circ }}\].
Option (c): The angle will be greater than \[{{90}^{\circ }}\] not exactly \[{{90}^{\circ }}\].
Option (d): The angle can be \[{{180}^{\circ }}\] only when ACB is a straight line.
Hence, option (b) is correct.

Note: The angle will be \[{{90}^{\circ }}\] only in the limiting case when the chord becomes diameter of the circle but in that case, both the segments will be equal and we will not get any minor or major segment.