
What Are Barkhausen Conditions For Oscillation?
Answer
415.2k+ views
Hint: An oscillator is a circuit, it produces a repeated, alternating waveform without any input. Oscillators work on the principle of positive feedback. A small fraction of the output of the amplifier is fed back to the input. so that the output generates continuously even though the input was removed. We are going to discuss the Barkhausen conditions for oscillation.
Complete answer:
For oscillator, the voltage gain of an amplifier is given by,
Where,
A-voltage gain without feedback
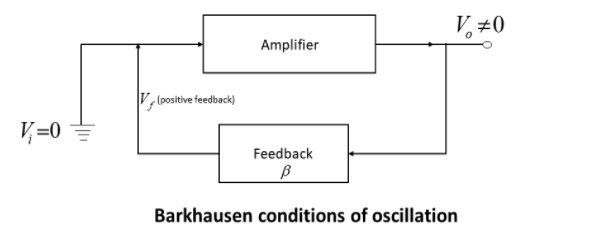
The firstBarkhausen condition is that the overall loop gain should be unity
By applying the above condition in the voltage gain of an amplifier,
We know that the voltage gain of the amplifier is
Where
If the voltage gain of the amplifier with feedback is infinity,
Therefore,
The second condition of Barkhausen conditions is that the total phase shift around loop gain should be
It is attained by positive feedback to the amplifier. The part of the output signal is fed back to the input signal which should be the same in phase with an input signal.
The two Barkhausen conditions of oscillation are (i) the overall loop gain should be unity and (ii) the total phase shift around loop gain should be
Note: From these conditions, the oscillator will produce sustained oscillations and will generate a sinusoidal signal. The Barkhausen condition is used in electronic circuits to find whether the circuit will oscillate or not. The feedback signal and the input signal are in phase for producing oscillations by giving positive feedback to the amplifier. If
Complete answer:
For oscillator, the voltage gain of an amplifier is given by,
Where,
A-voltage gain without feedback

The firstBarkhausen condition is that the overall loop gain should be unity
By applying the above condition in the voltage gain of an amplifier,
We know that the voltage gain of the amplifier is
Where
If the voltage gain of the amplifier with feedback is infinity,
Therefore,
The second condition of Barkhausen conditions is that the total phase shift around loop gain should be
It is attained by positive feedback to the amplifier. The part of the output signal is fed back to the input signal which should be the same in phase with an input signal.
The two Barkhausen conditions of oscillation are (i) the overall loop gain should be unity and (ii) the total phase shift around loop gain should be
Note: From these conditions, the oscillator will produce sustained oscillations and will generate a sinusoidal signal. The Barkhausen condition is used in electronic circuits to find whether the circuit will oscillate or not. The feedback signal and the input signal are in phase for producing oscillations by giving positive feedback to the amplifier. If
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




