
As we observed from the top of a 75 m lighthouse from the sea level, the angle of depression of 2 ships
Answer
527.7k+ views
Hint: Draw a rough figure of the lighthouse and 2 boats and mark the angle of depression. Consider the triangle where
Complete step-by-step answer:
Given is the height of the lighthouse = 75 m.
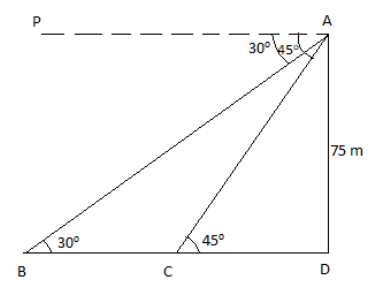
Hence from the figure, we can say that, AD = 75 m
Let the angle of depression of first ship be
The angle of depression of second ship will be
We need to find the distance between the 2 ships, i.e. we need to find the length of BC.
From the figure, we can understand that lines PA and BD are parallel, i.e.
Thus AB and AC are transversals.
We can say that,
where both these angles are alternate angles as
The lighthouse is perpendicular to the ground, i.e.
From the figure, let us consider
We know AD = 75 m, let’s find CD and by trigonometric table
Now let us consider
We know AD = 75 m, CD = 75 cm, and from trigonometric table,
Cross multiply and find the value of BC.
Thus we got the distance between 2 ships as
Hence, distance between 2 ships
Note:
We have been given the angle of depression which is the angle from the top of the lighthouse to the bottom 2 ships. But as the lines formed are parallel they become alternate angles. So the elevation from the 2 boats to the top of the lighthouse will become
Complete step-by-step answer:
Given is the height of the lighthouse = 75 m.

Hence from the figure, we can say that, AD = 75 m
Let the angle of depression of first ship be
The angle of depression of second ship will be
We need to find the distance between the 2 ships, i.e. we need to find the length of BC.
From the figure, we can understand that lines PA and BD are parallel, i.e.
Thus AB and AC are transversals.
We can say that,
where both these angles are alternate angles as
The lighthouse is perpendicular to the ground, i.e.
From the figure, let us consider
We know AD = 75 m, let’s find CD and by trigonometric table
Now let us consider
We know AD = 75 m, CD = 75 cm, and from trigonometric table,
Cross multiply and find the value of BC.
Thus we got the distance between 2 ships as
Hence, distance between 2 ships
Note:
We have been given the angle of depression which is the angle from the top of the lighthouse to the bottom 2 ships. But as the lines formed are parallel they become alternate angles. So the elevation from the 2 boats to the top of the lighthouse will become
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




