
Assertion: Time taken by a particle in SHM to move from
Reason: Corresponding angles in the reference circle are the same in the given time interval.
A. If both Assertion and Reason are true and the Reason is the correct explanation of the Assertion.
B. If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
C. If Assertion is true, but the Reason is false.
D. If Assertion is false but the Reason is true.
Answer
425.1k+ views
Hint: SHM stands for Simple Harmonic Motion .It is a type of oscillating motion. An object oscillates sinusoidally about an equilibrium point during a SHM. Here, we need to find the time interval for both intervals using the standard equation of SHM. We also need to check the truth value of the reason statement and check if it is a good explanation for the assertion statement.
Formula used:
The motion of SHM can be described as:
where,
Complete step by step answer:
Let us consider the assertion statement. Given that, at the instant
At the instant say
At the instant say
Now we know the displacement equation is given by
Now, let us substitute the conditions at time
Substituting the obtained value in the standard equation,
Now, when the particle is at point
Now, we know that angular frequency can be written as
Now, when the particle is at point
Now, we know that angular frequency can be written as
Now, the time taken by the particle to reach from
Now, the time taken by the particle to reach from
Hence, the time taken in both intervals is the same. Hence, the assertion statement is correct.
Now, let us consider the reason statement.
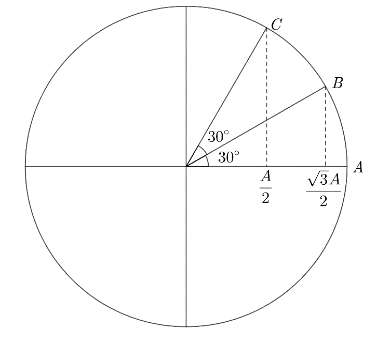
The reference circle in SHM is a circle with radius equal to the amplitude as shown above
We know that the time interval for both the conditions given here is the same. We can say from the figure that when we plot the given conditions on the reference circle, the corresponding angle rotation is also equal. Hence, the reason statement is correct and a correct explanation for the assertion statement.
Hence, the correct option is A.
Note: SHM or Simple Harmonic Motion uses a lot of new concepts and words that you people are familiar with but the basic concepts are the same so if you get stuck at any point of time keep in mind the things you studied in lower classes.
Formula used:
The motion of SHM can be described as:
where,
Complete step by step answer:
Let us consider the assertion statement. Given that, at the instant
At the instant say
At the instant say
Now we know the displacement equation is given by
Now, let us substitute the conditions at time
Substituting the obtained value in the standard equation,
Now, when the particle is at point
Now, we know that angular frequency can be written as
Now, when the particle is at point
Now, we know that angular frequency can be written as
Now, the time taken by the particle to reach from
Now, the time taken by the particle to reach from
Hence, the time taken in both intervals is the same. Hence, the assertion statement is correct.
Now, let us consider the reason statement.

The reference circle in SHM is a circle with radius equal to the amplitude as shown above
We know that the time interval for both the conditions given here is the same. We can say from the figure that when we plot the given conditions on the reference circle, the corresponding angle rotation is also equal. Hence, the reason statement is correct and a correct explanation for the assertion statement.
Hence, the correct option is A.
Note: SHM or Simple Harmonic Motion uses a lot of new concepts and words that you people are familiar with but the basic concepts are the same so if you get stuck at any point of time keep in mind the things you studied in lower classes.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

The reason why India adopted the policy of nonalignment class 11 social science CBSE

List out the uses of ethanoic acid class 11 chemistry CBSE

The process in which gas transform into solid is called class 11 chemistry CBSE

The heart of a computer is A CPU B Memory C Input D class 11 computers CBSE




