
At what angle diagonals of a square intersect each other ?
Answer
400.8k+ views
Hint: Here in this question, we need to find the angle in intersection point of two diagonals in the square. For this first we need to draw a shape of square and two diagonals then we can measure or identify an angle made when two diagonals meet or intersect the point of two diagonals.
Complete step-by-step solution:
Square is a regular quadrilateral or four-sided polygon, which has all the four sides of equal length in measurement and all four angles are also equal. The angles at the corner of the square are at right-angle or equal to 90-degrees.
Diagonal is a straight line connecting two non-adjacent angles or vertices of a polygon or shapes of geometry.
In square, the length of the diagonals are equal and bisect each other at
Now consider the Square ABCD all sides of the square are equal length i.e., AB = BC = CD = AD.
In square ABCD, AD and BC are the diagonals and ‘O’ be the intersection point of two diagonals AD and BC.
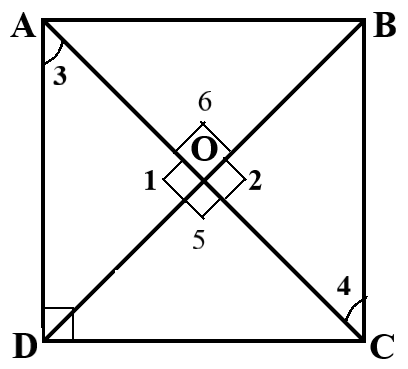
In square
Consider triangles
In
In
In
In line AC
By the above information
Divide both side by 2, then we get
Therefore, at
Note: While solving these type of questions, we have to know the some basic properties, Axiom and postulates of triangle like when two triangles are congruent all sides and angles of two triangles should be equal, when if two triangles are similar the corresponding sides are in proportion and the corresponding angles are congruent and know about postulates like SAS, ASA, AAA etc…
Complete step-by-step solution:
Square is a regular quadrilateral or four-sided polygon, which has all the four sides of equal length in measurement and all four angles are also equal. The angles at the corner of the square are at right-angle or equal to 90-degrees.
Diagonal is a straight line connecting two non-adjacent angles or vertices of a polygon or shapes of geometry.
In square, the length of the diagonals are equal and bisect each other at
Now consider the Square ABCD all sides of the square are equal length i.e., AB = BC = CD = AD.
In square ABCD, AD and BC are the diagonals and ‘O’ be the intersection point of two diagonals AD and BC.

In square
Consider triangles
In
In
In
In line AC
By the above information
Divide both side by 2, then we get
Therefore, at
Note: While solving these type of questions, we have to know the some basic properties, Axiom and postulates of triangle like when two triangles are congruent all sides and angles of two triangles should be equal, when if two triangles are similar the corresponding sides are in proportion and the corresponding angles are congruent and know about postulates like SAS, ASA, AAA etc…
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE




