
Calculate tensions

Answer
480.9k+ views
Hint: The tension in a particular string is affected by the weight of the boxes below it, whether immediate or not. Since the boxes accelerate upward, the force in the upward direction acting on a particular box is greater than the force acting in the downward direction. So in the equation of motion of each body we need to substitute the values and then we will get the answer.
Formula used: In this solution we will be using the following formulae;
Complete step by step solution:
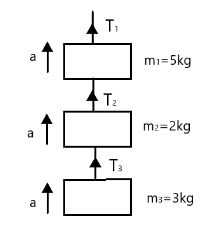
In the diagram, the weight of all boxes acts on string
To find the tension in each string we analyse the dynamics of each box. For simplicity, let’s start from the bottom
For the bottom box, the tension
Now, since the weight of a body is given as
Making
Inserting all known values
For the box of mass
The tension
Therefore, we have
Inserting values, we have
Similarly for top box we have
Inserting values, we have
Hence,
Note:
To avoid confusion, the tension
Formula used: In this solution we will be using the following formulae;
Complete step by step solution:
In the diagram, the weight of all boxes acts on string
To find the tension in each string we analyse the dynamics of each box. For simplicity, let’s start from the bottom
For the bottom box, the tension
Now, since the weight of a body is given as
Making
Inserting all known values
For the box of mass
The tension
Therefore, we have
Inserting values, we have
Similarly for top box we have
Inserting values, we have
Hence,
Note:
To avoid confusion, the tension
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




