Question

Calculate the area of the shaded region in the figure, where quadrilateral ABCD is a square with side 8 cm each. $\left( \pi =3.14 \right)$

a) 36.48 $c{{m}^{2}}$
b) 25.40 $c{{m}^{2}}$
c) 15 $c{{m}^{2}}$
d) 65 $c{{m}^{2}}$

Answer 1

Hint: When you observe closely you can see 2 quadrants of circle overlapping to form a square. For quadrants the two perpendicular lines are radius. So, they will be equal. If we add the area of 2 quadrants then we get the area of the square with extra term as there is overlapping between the quadrants. So, if you subtract the area of the triangle formed by the diagonal of the square. You will get the half of the required shaded area. So, now multiply it by 2 to get the required area. By the basic knowledge of geometry, we can say the area of following:
Square $={{S}^{2}}$ , triangle $=\dfrac{1}{2}bh$ , quadrant $=\dfrac{1}{4}\pi {{r}^{2}}$

Complete step-by-step answer:
Square: Square is a 2-dimensional figure with all sides equal. The diagonal of the square divides it into 2 triangles.
Quadrant: The $\dfrac{1}{4}th$ part of the circle is called a quadrant. The radius of this quadrant makes $90{}^\circ $ with each other.

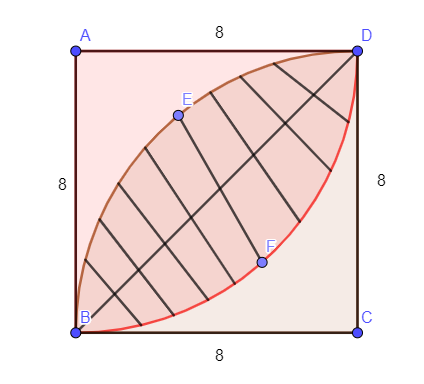
Quadrant ABFD is the quadrant we consider for our solution. We also consider triangle ABD for subtracting purposes.
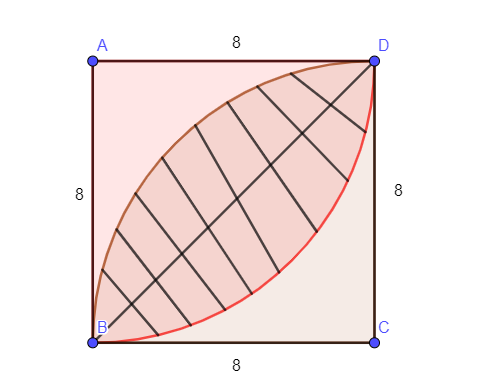
As you can see in the figure, the shaded area is divided into 2 halves by the diagonals of square, BD.
So, if we subtract the area of the triangle from the quadrant, we get half of the required area. So, we will multiply by 2.
Let us assume the area of the shaded region be S.
By above conditions we can say that value of S will be:
$S=2\left( \text{area of ABFD }-\text{ area of ABD} \right)$
Area of ABFD ; By basic knowledge of geometry
Area of quadrant $=\dfrac{\pi {{r}^{2}}}{4}$ , here, $r=8$
$=\dfrac{\pi {{\left( 8 \right)}^{2}}}{4}$
By solving we get ABFD $=50.24c{{m}^{2}}$
Area of triangle ABD; base $=8cm$ height $=8cm$
Area $=\dfrac{1}{2}\times b\times h=\dfrac{1}{2}\times 64$
Area of ABD $=32c{{m}^{2}}$
By substituting into equation of S, we get value as:
$S=2\left( ABFD-ABD \right)=2\left( 50.24-32 \right)c{{m}^{2}}$
By simplifying the above equation, we get value of A as:
$S=36.48c{{m}^{2}}$
Therefore, the area of the shaded region is 36.48 $c{{m}^{2}}$ .
Option (a) is correct.

Note: The idea of multiplying two is crucial. Alternately you can apply the same on other quadrants and add both to get the result. Whenever you see an unknown geometrical figure, try to divide it into combinations of known figures so that you can apply area formulae which you know. Here it is a combination of triangles inside a sector of a circle. It is important that you view it in that sense.