
Calculate the equivalent mass of
Answer
417.9k+ views
Hint: The given reaction between hydrochloric acid and potassium dichromate is a redox reaction. The equivalent mass of hydrogen chloride can be calculated by knowing its molecular mass and its
Complete answer:
The
In the reaction between hydrochloric acid and potassium dichromate, the dichromate ion is being reduced to
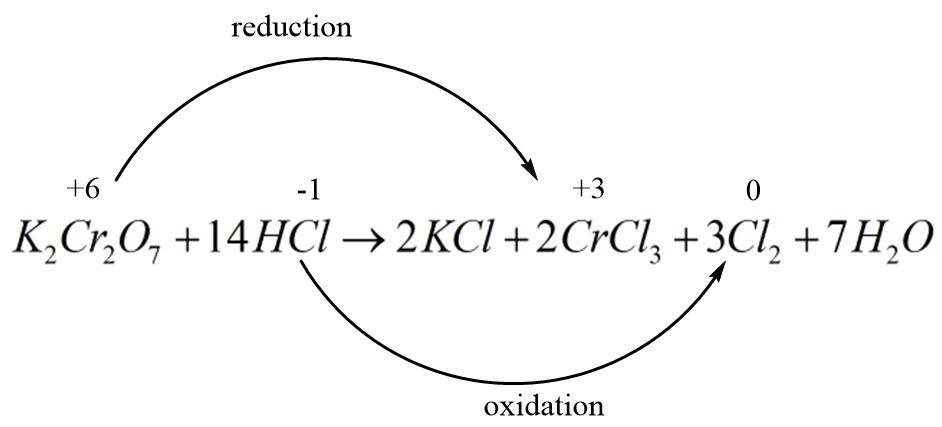
In order to find out the
a.Oxidation
b.Reduction
The electrons gained and lost in a balanced redox reaction must be equal. Hence the oxidation reaction is multiplied by three and the reduction reaction is multiplied by two.
Thus there are six electrons being involved in the redox reaction for fourteen
The formula of
Putting the number of involved electrons as six and number of involved molecules as fourteen in the above formula we get,
The equivalent mass can be calculated by finding out the ratio of the molar mass and
Which when simplified gives,
Hence, the equivalent mass of
Note:
The oxidation numbers assigned to atoms in the chemical equation are hypothetical numbers that represent the number of electrons donated by or accepted by that atom in making bonds with the atoms present in the molecule.
Complete answer:
The
In the reaction between hydrochloric acid and potassium dichromate, the dichromate ion is being reduced to

In order to find out the
a.Oxidation
b.Reduction
The electrons gained and lost in a balanced redox reaction must be equal. Hence the oxidation reaction is multiplied by three and the reduction reaction is multiplied by two.
Thus there are six electrons being involved in the redox reaction for fourteen
The formula of
Putting the number of involved electrons as six and number of involved molecules as fourteen in the above formula we get,
The equivalent mass can be calculated by finding out the ratio of the molar mass and
Which when simplified gives,
Hence, the equivalent mass of
Note:
The oxidation numbers assigned to atoms in the chemical equation are hypothetical numbers that represent the number of electrons donated by or accepted by that atom in making bonds with the atoms present in the molecule.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




