
Calculate the following in a triangle ABC, D is the midpoint of AB and E is the midpoint of AC.
i)DE if BC=8cm
ii)
b)If
Answer
494.1k+ views
Hint: We prove the similarity of triangles ADE and ABC in part(a). We use the ratio of sides to get DE. We use the equality of corresponding angles to get
Complete step-by-step solution:
(a) We have the triangle ABC , D is the midpoint o f AB and E is the midpoint of AC ,
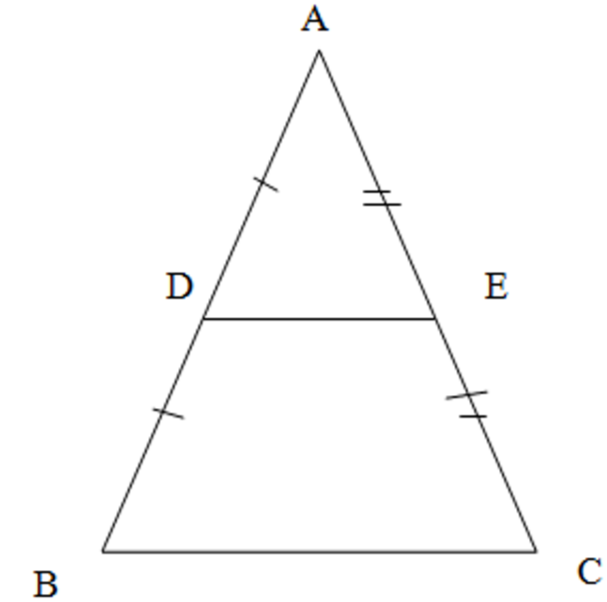
We have joined DE. . We know that lie joining the midpoints two sides will be parallel to the other side. So
We observe the triangles ADE and ABC. We have corresponding angles formed by the line AB cutting the parallel lines DE and BC . They will be equal. So we have
So the sides will be in equal ratio which means
(i)We are given that BC=8cm. So we find
(ii) We are given
(b) We are given
We proceed by replacing the composite numbers 9 and 27 by their prime factorization.
We use the formula
We use the formula
The above result is true when for integral values of
Note: We need to take care of confusion of similarity from congruence which is the equality of angles and sides of two different triangles. We can only find a non-integral solution when the base of the exponents
Complete step-by-step solution:
(a) We have the triangle ABC , D is the midpoint o f AB and E is the midpoint of AC ,

We have joined DE. . We know that lie joining the midpoints two sides will be parallel to the other side. So
We observe the triangles ADE and ABC. We have corresponding angles formed by the line AB cutting the parallel lines DE and BC . They will be equal. So we have
So the sides will be in equal ratio which means
(i)We are given that BC=8cm. So we find
(ii) We are given
(b) We are given
We proceed by replacing the composite numbers 9 and 27 by their prime factorization.
We use the formula
We use the formula
The above result is true when for integral values of
Note: We need to take care of confusion of similarity from congruence which is the equality of angles and sides of two different triangles. We can only find a non-integral solution when the base of the exponents
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

What are the public facilities provided by the government? Also explain each facility

Discuss the main reasons for poverty in India

What is the past participle of wear Is it worn or class 10 english CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE

Compare the advantages and disadvantages of multipurpose class 10 social science CBSE




